On Pseudo Weakly J-clean Rings
2016-12-16胡小美陈焕艮
胡小美, 陈焕艮
(杭州师范大学理学院, 浙江 杭州 310036)
On Pseudo Weakly J-clean Rings
A ringRis called a pseudo weakly J-clean ring if every elementa∈Rcan be written in the form ofa=e+w+(1-e)Raora=-e+w+(1-e)Rawhereeis an idempotent andwbelongs to the Jacobson radical. This paper explores various properties of pseudo weakly J-clean rings. A ringRis pseudo weakly J-clean if and only ifR[[x]], Hurwitz series ringH(R), trivial extensionT(R,M) andS(R,σ) are pseudo weakly J-clean, respectively. Furthermore, it proves that the following are equivalent, for anyn∈N,Sn(R) is pseudo J-clean, for anyn∈N,R[x]/(xn) is pseudo weakly J-clean, where (xn) is the ideal generated byxn. In particular, it characterizeS=R[D,C] is pseudo weakly J-clean under certain conditions. Also it shows that 2 is a unit inR, thenRis pseudo J-clean if and only ifRC2is pseudo J-clean.
pseudo weakly J-clean ring; Hurwitz series ring;S=R[D,C] ring; group ring; Jacobson radical; idempotent
1 Introduction
Following Qua[1], we call a ringRis pseudo weakly clean if every elementa∈Rcan be written in the form ofa=e+u+(1-e)Raora=-e+u+(1-e)Rawhereeis an idempotent anduis a unit. On the other hand, J-clean rings are studied by Chen[2]. A ringRis called a J-clean ring if every elementa∈Rcan be written in the form ofa=e+jwhereeis an idempotent andjbelongs to Jacobson radical. If we add an extra condition thatej=jeon the above definition it is called a strongly J-clean ring. Now, we combine pseudo weakly rings and weakly J-clean rings together and consider a new type of rings. We call a ringRis pseudo J-clean if every elementa∈Rcan be written in the form ofa=e+w+(1-e)Rawhereeis an idempotent andwbelongs to Jacobson radical and an elementa∈Ris said to be pseudo weakly J-clean if existe∈Id(R) andw∈J(R) such thata=e+w+(1-e)Raora=-e+w+(1-e)Ra.
We explore various properties of pseudo weakly J-clean rings, we proves that the direct product ringR=∏k∈IRkis pseudo weakly J-clean if and only if eachRkis pseudo weakly clean and at most oneRkis not a pseudo J-clean ring. A ringRis pseudo weakly J-clean if and only ifR[[x]], Hurwitz series ringH(R), trivial extensionT(R,M) andS(R,σ) are pseudo weakly J-clean, respectively. Furthermore, we prove that the following are equivalent: the ringTn(R) ofn×nupper triangular matrices overRis pseudo J-clean;QM2(R) is pseudo J-clean; For anyn∈N,Sn(R) is pseudo J-clean; For anyn∈N,R[x]/(xn) is pseudo weakly J-clean, where (xn) is the ideal generated byxn. In particular, the following conditions are equivalent:S=R[D,C] is pseudo weakly J-clean;DandCis pseudo weakly J-clean;S′=R{D,C} is pseudo weakly J-clean. Also we show that 2 is a unit inR, thenRis pseudo J-clean if and onlyRC2is pseudo J-clean.
Throughout this paper, the rings that we discussed are associative rings with an identity.Id(R) denotes the idempotents ofR,J(R) denotes the Jacobson radical ofR,U(R) denotes the unit ofRand we useTn(R) to stand for the ring of alln×nupper triangular matrices over a ringR.
2 Equivalent Characterizations
Definition 1 A ringRis called a pseudo weakly J-clean ring if every elementa∈Rcan be written in the form ofa=e+w+(1-e)Raora=-e+w+(1-e)Rawheree∈Id(R) andw∈J(R).
The ringRis said to be pseudo weakly J-clean if all of its elements are pseudo weakly J-clean.
Theorem 1 Every homomorphic image of a pseudo weakly J-clean ring is pseudo weakly J-clean.
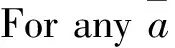
WecallaringispseudoJ-cleanifthereexistanidempotenteandaJacobsonradicalwinRsuchthata=e+w+(1-e)Raforanya∈R.
Proposition1LetRbearing.Thenthefollowingconditionsareequivalent:
1)RisapseudoJ-clean;
2)Everyelementx∈Rhastheformx=-e+w+(1-e)rxwherew∈J(R), e∈Id(R),andr∈R.
Proof1) 2).Letx∈R.SinceRispseudoJ-clean,wehave-x=w′+e+(1-e)r(-x),herew′∈J(R), e∈Id(R)andr∈R.Thenx=-w′-e+(1-e)rx.Set-w′=w,wegetx=w-e+(1-e)rx.
2) 1).Letx∈R.Then-x=-e+w+(1-e)r(-x)forsomew∈J(R), e∈Id(R)andr∈R.Wemusthavex=-w+e+(1-e)rx,because-w∈J(R),itshowsthatxispseudoJ-clean.
Theorem2ThedirectproductringR=∏k∈IRkispseudoweaklyJ-cleanifandonlyifeachRkispseudoweaklyJ-cleanandatmostoneRkisnotapseudoJ-cleanring.
Proof( ):SupposethatR=∏k∈IRkispseudoweaklyJ-clean.ByTheorem1,wecanknowthateachRkispseudoweaklyJ-cleanbecauseeachRkisahomomorphicimageofR.SupposethatRiandRj(i≠j)arenotpseudoJ-clean.SinceRiisnotpseudoJ-clean,thenbyProposition1,thereexistsxi∈Risuchthatxi≠w-e+(1-e)rxiforanyw∈J(Ri), e∈Id(Ri)andr∈Ri.ButRiisapseudoweaklyJ-cleanring,wemusthavexi=wi+ei+(1-ei)rixiforsomewi∈J(Ri), ei∈Id(Ri)andri∈Ri.NowsinceRjisnotpseudoJ-cleanbutpseudoweaklyJ-clean,thereisanxj∈Rj,wehavexj=wj-ej+(1-ej)rjxjforsomewj∈J(Rj), ej∈Id(Rj)andrj∈Rjbutxj≠w+e+(1-e)rxjforanyw∈J(Rj), e∈Id(Rj)andr∈Rj.Lety=(yk)∈Rsuchthat
Thenwegety≠w±e+(1-e)ryforanyw∈J(R), e∈Id(R)andr∈R,whichimpliesthatyisnotpseudoweaklyJ-clean;whichcontradictstheassumptionthatRispseudowealkJ-clean.Hence,wecangetatmostoneRkisnotapseudoJ-cleanring.
(⟸):IfeveryRiispseudoJ-clean,thisissimilartotheproofof[3,Proposition2.3]thatR=∏k∈IRkisalsopseudoJ-clean,soitispseudoweaklyJ-clean.SupposethatRi0ispseudoweaklyJ-cleanbutnotpseudoJ-cleanandalltheotherRiarepseudoJ-clean.Letx=(xi)∈R=∏k∈IRk.Thenxi0=wi0±ei0+(1-e)ri0xi0foreachxi0∈Ri0,herewi0∈J(Ri0), ei0∈Id(Ri0)andri0∈Ri0.Ifxi0=wi0+ei0+(1-e)ri0xi0,thenfori≠i0andRiispseudoJ-clean,wemusthavexi=wi+ei+(1-ei)rixiwherewi∈J(Ri), ei∈Id(Ri)andri∈Ri.Ifxi0=wi0-ei0+(1-e)ri0xi0,thenfori≠i0andRiispseudoJ-clean,thenbyProposition1thatwecanwritexi=wi-ei+(1-ei)rixiwherewi∈J(Ri), ei∈Id(Ri)andri∈Ri.Hence, x=w+e+(1-e)rxorx=w-e+(1-e)rxwherew=w(j)∈U(R), e=(ej)∈Id(R)andr=(rj)∈R.Therefore, xispseudoweaklyJ-clean.Thiscompletestheproof.
Corollary1ThedirectproductringR=∏k∈IRkispseudoJ-cleanifandonlyifeachRkispseudoJ-clean.
ProofItisobvious.
Theorem3LetRbearing.ThenRispseudoweaklyJ-cleanifandonlyifR[[x]]ispseudoweaklyJ-clean.
ProofSupposethatRispseudoweaklyJ-clean,foranya∈R,wehavea=e+w+(1-e)r(x)ora=-e+w+(1-e)r(x).Ifa=e+w+(1-e)r(x),then
f(x)= a0+a1x+a2x2+…=e+w+(1-e)ra0+(a1x+a2x2+…)=
e+w+(a1x+a2x2+…)-(1-e)r(a1x+a2x2+…)+(1-e)rf(x)=
e+α(x)+(1-e)rf(x).
Similarly,ifa=-e+w+(1-e)r(x),thenf(x)=-e+α(x)+(1-e)rf(x).Itiseasytoknowα(x)∈J(R[[x]]),hence, R[[x]]ispseudoweaklyclean.
Conversely,supposethatR[[x]]ispseudoweaklyJ-clean.ThenitfollowsbytheisomorphismR≅R[[x]]/(x)andTheorem1thatRispseudoweaklyJ-clean.
Corollary2LetRbearing. R[[x1,…, xn]]ispseudoweaklyJ-cleanifandonlyifRispseudoweaklyJ-clean.
ProofByinductionandTheorem3.
Proposition2LetRbearing.ThenthepolynomialringR[x]isneverpseudoweaklyJ-cleanring.
ProofSupposethatx∈R[x]ispseudoweaklyJ-clean,thenx=e+w+(1-e)rxorx=-e+w+(1-e)rxforsomew∈J(R[x]), e∈Id(R[x])andr∈R[x].Wemaywritee=e0+e1x+…+enxnandw=w0+w1x+…+wnxnwhere(en, wn)≠(0, 0).Then
x=w+e+rx-erx=(w0+w1x+…+wnxn)+(e0+e1x+…+enxn)+rx-(e0+e1x+…+enxn)rx
or
x=w-e+rx-erx=(w0+wx1x+…+wnxn)-(e0+e1x+…+enxn)+rx-(e0+e1x+…+enxn)rx.

1=x(1+w)-1=b0x+b1x2+b2x3+…+blxl+1.




Lemma1[4]LetRbearing.ThentheHurwitzseriesx=(x0, x1, x2,…)isaunitinH(R)ifandonlyifx0isaunitinR.
Theorem4LetRbearing.ThentheHurwitzseriesx=(x0, x1, x2,…)isintheJacobsonradicalofH(R)ifandonlyifx0isintheJacobsonradicalinR.
ProofLetx=(xn)∈H(R), r=(rn)∈H(R).Ifx=(xn)∈J(H(R)),then1-ax=(1-r0x0,…)∈U(H(R)).ItfollowsfromtheLemma1,wecanknow1-r0x0∈U(R).Therefore, x0∈J(R).Conversely,ifx0∈J(R),foranyr∈R,wehave1-rx0∈U(R),therefore, 1-r0x0∈U(R).ItfollowsfromtheLemma1,wecanknow1-ax=(1-r0x0,…)∈U(H(R)).Hence, x=(xn)∈J(H(R)).
Theorem5LetRbearing.ThenH(R)ispseudoweaklyJ-cleanifandonlyifRispseudoweaklyJ-clean.
ProofByTheorem1,everyhomomorphicimageofapseudoweaklyJ-cleanringispseudoweaklyJ-clean,soR≅H(R)/kerφispseudoweaklyJ-clean,whereφ:H(R)→R.
Conversely,supposethatRispseudoweaklyJ-clean.Letx=(xn)∈H(R).Thenx0∈R.Hence, x0=±e+w′+(1-e)rx0fore∈Id(R)andw′∈J(R).Thusx=λR(e)+w+λR[(1-e)rx]=(e, 0, 0,…)+w+((1-e)rx, 0, 0,…)orx=-λR(e)+w+λR[(1-e)rx]=-(e, 0, 0,…)+w+((1-e)rx, 0, 0,…),wherew∈J(H(R)).

Proposition3LetRbeapseudoJ-cleanring.Thenthefollowingstatementshold:
1)TheringTn(R)ofn×nuppertriangularmatricesoverRispseudoJ-clean;
2)QM2(R)ispseudoJ-clean;
4)For anyn∈N,R[x]/(xn) is pseudo J-clean, where (xn) is the ideal generated byxn.

3)Theproofissimilartothatof1).
4)NotethatR[x]/(xn)≅Sn(R),weobtaintheresultby3).
GivenaringRanda(R, R)-bimoduleM,thetrivialextensionofRbyMistheringT(R, M)=R⊕Mwiththeusualadditionandthefollowingmultiplication: (r1, m1)(r2, m2)=(r1r2, r1m2+m1r2).AsweallknowJ(R×M)={(r, m)|r∈J(R), m∈RMR}.
Proposition4LetRbearing.ThenRispseudoweaklyJ-cleanifandonlyifT(R, M)ispseudoweaklyJ-clean.
ProofIfRisweaklyJ-clean,forany(x, m)∈T(R, M)sinceRisweaklyJ-clean, x=e+w+(1-e)rxorx=-e+w+(1-e)rx,wheree∈Id(R), w∈J(R).Wehave(x, m)=(e+w+(1-e)rx, m)or(x, m)=(-e+w+(1-e)rx, m).Hence(x, m)=(e, 0)+(w, m)+((1-e)rx, 0)or(x, m)=-(e, 0)+(w, m)+((1-e)rx, 0)where(e, 0)∈Id(T(R, M))and(w, m)∈J(T(R, M)),soT(R, M)ispseudoweaklyJ-clean.
Conversely,ifT(R, M)ispseudoweaklyJ-clean,foranyx∈R, (x, 0)∈T(R, M),wehave(x, 0)=(e, *)+(w, *)+((1-e)rx, 0)or(r, 0)=-(e, *)+(w, *)+((1-e)rx, 0)where(e, *)2=(e2, *)=(e, *)and(w, *)∈J(T(R, M))thatisx=e+w+(1-e)rxorx=-e+w+(1-e)rxwheree∈Id(R)andw∈J(R),soRispseudoJ-weakly.

Theorem 6 LetRbe a ring. ThenRis pseudo weakly J-clean if and only ifS(R,σ) is pseudo weakly J-clean.


3 Related Rings
Inthissection,wefurtherconsiderpseudoweakJ-cleannessofvariousrelatedrings.WenowconsidertheconnectionofpseudoweakJ-cleannessandweakJ-cleanness.


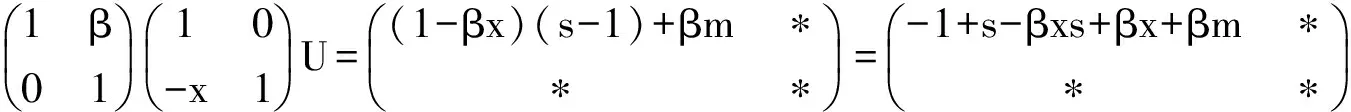
Letu=α+βγa=-1+s-βxs+βx+βm∈-1+J(R).Thensubstitutingα=u-βγaintotheequationαa=fα+1,weget
ua-βγa2= f(u-βγa)+1=fu+1 u-1(ua-βγa2)=u-1(fu+1)
a-u-1βγa2=u-1fu+u-1a-u-1fu-u-1=u-1βγa2.
Wesetu-1=-1+w′, w′∈J(R),itfollowsthat
a-u-1fu-(-1+w′)-u-1βγ= u-1βγ(a-1)(a+1)
a+1-u-1fu-(w′+u-1βγ)=u-1βγ(a-1)(a+1).
Fromtherelationfβ=0,wecanknowβ=eβ.Wehave
a+1-u-1fu-(w′+u-1βγ)= u-1eβγ(a-1)(a+1)=u-1eu(u-1βγ)(a-1)(a+1)=
(1-u-1fu)(u-1βγ)(a-1)(a+1)∈(1-u-1fu)R(a+1).
Setu-1fu=g1∈Id(R), w1=w′+u-1βγ∈J(R),andweknowb=a+1,sob-g1-w1∈(1-g1)Rb,asdesired.
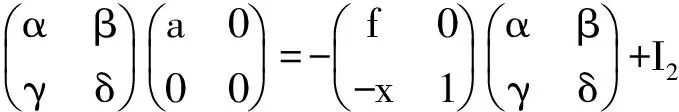
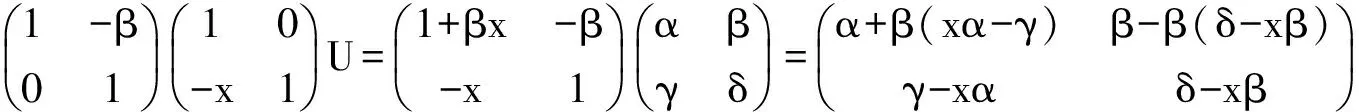

Letv=α+βγaandbyusingsimilartothoseabove,wecanknowv=α+βγa=-1+s+βxs-βx-βm∈-1+J(R)andwesetv-1=-1+w″, w″∈J(R),itfollowsthat
a+v-1fv-(-1+w″)-v-1βγ= v-1βγ(a-1)(a+1)
a+1+v-1fv-(w″+v-1βγ)=v-1βγ(a-1)(a+1).
Fromtherelationfβ=0,wecanknowβ=eβ.Wehave
a+1+v-1fv-(w″+v-1βγ)= v-1eβγ(a-1)(a+1)=v-1ev(v-1βγ)(a-1)(a+1)=
(1-v-1fv)(v-1βγ)(a-1)(a+1)∈(1-v-1fv)R(a+1).
Setv-1fv=g2∈Id(R), w2=w″+v-1βγ∈J(R),andweknowb=a+1,sob+g2-w2∈(1-g2)Rb,asdesired.
LetDbearing, CbeasubringofDand1D∈C,write:
S=R[D, C]={(d1,…, dn, c, c,…)|di∈D, c∈C, n≥1},
S′=R{D, C}={(d1,…, dn, cn+1, cn+2,…)|di∈D, cj∈C, n≥1}.
Here, the addition and multiplication are defined componentwise, bothS=R[D,C] andS=R{D,C} are rings with identities.
Lemma 2 1)J(S)=R[J(D),J(D)∩J(C)];
2)J(S′)=R{J(D),J(D)∩J(C)}.
Proof See [5, Proposition 2.1.14].
Theorem 8 LetS=R[D,C]. Then the following conditions are equivalent:
1)S=R[D,C] is pseudo weakly J-clean;
2)Dis pseudo weakly J-clean and for anya∈C, there existw∈J(D)∩J(C),e∈Id(C), such thata-w±e∈(1-e)Ca;
3)S′=R{D,C} is pseudo weakly J-clean.

3)⟺2).Itissimilarto1)⟺2).
GivenagroupGandaringA,thegroupringR=AG,consistsofallfunctionsr:G→Awithfinitesupport.Thesupportofris{g∈G|r(g)≠0}. Risendowedwithringoperationbydefining:
Let us verify thatR(0, 1, -, +, ·) is in fact a ring.
We first consider some cases where a group ring is isomorphic (as a ring) to a direct product of copies of the coefficient ring.
Proposition 5 LetRbe a ring and let 2 be a unit inR. ThenRis pseudo J-clean if and only ifRC2is pseudo weakly J-clean.
Proof LetC2={x|x2=1} and defineφ:RC2→R×Rbyφ(a+bx)=(a+b,a-b) wherea,b∈R. Thenψis a ring homomorphism. Since 2 is a unit inR, we have thatψis bijective. Therefore,RC2≅R×R. IfRis pseudo J-clean, it follows by Corollary 1 thatRC2≅R×Ris pseudo weakly J-clean; Conversely, ifRC2≅R×Ris pseudo weakly J-clean, it follows by Theorem 1 thatR×Ris pseudo weakly J-clean. Therefore,Ris pseudo J-clean by Theorem 2.
Proposition 6 LetRbe a ring and let 2 be a unit inR. ThenRis pseudo J-clean if and only ifRC2is pseudo J-clean.
Proof As in the proof of Proposition 5, it may be shown thatRC2≅R×R. It then follows by Corollary 1 thatRis pseudo J-clean if and only ifRC2is pseudo J-clean.
By Proposition 5 and Proposition 6 we have the following.
Corollary 3 LetRbe a ring and let 2 be a unit inR. Then the following are equivalent:
1)Ris pseudo J-clean;
2)RC2is pseudo weakly J-clean;
3)RC2is pseudo J-clean.
Proposition 7 LetRbe a ring and let 2 be a unit inR. Then the following are equivalent:
1)Ris pseudo J-clean;

3) 2). It is obvious.
[1] QUA K T. Weakly clean and related rings[D]. Kuala Lumpur: The University of Malaya,2015.
[2] CHEN H Y. On strongly J-clean rings[J]. Comm Algebra,2010,38(10):3790-3804.
[3] STER J. Weakly clean rings[J]. J Algebra,2014,401(401):1-12.
[4] KEIGHER W F. On the ring of Hurwize series[J]. Comm Algebra,1997,25(25):1845-1859.
[5] CHENG G P. The structure of ringR[D,C] and its characterizations[D]. Nanjing: Nanjing University,2006.
HU Xiaomei, CHEN Huanyin
(School of Science, Hangzhou Normal University, Hangzhou 310036, China)
一个环R叫做 pseudo weakly J-clean 环,如果R中的每一个元素都可以写成a=e+w+(1-e)Ra或a=-e+w+(1-e)Ra的形式,其中e是幂等元,w属于Jacobson根.文章探究了pseudo weakly J-clean环的各种性质.环R是pseudo weakly J-clean环当且仅当幂级数环R[[x]], Hurwitz级数环H(R),平凡扩张T(R,M)和S(R,σ)分别是pseudo weakly J-clean环.更进一步证明以下几点是等价的:任意的n∈N,Sn(R)是 pseudo J-clean;任意的n∈N,R[x]/(xn)是pseudo J-clean, (xn)是由xn生成的理想.特别的,阐述了在某种条件下S=R[D,C]是pseudo weakly J-clean;并且得出结论:当2是R中的可逆元时,R是pseudo J-clean当且仅当群环RC2是pseudo J-clean.
pseudo weakly J-clean环;Hurwitz级数环;S=R[D,C]环;群环;Jacobson根;幂等元
关于Pseudo Weakly J-clean环
胡小美, 陈焕艮
(杭州师范大学理学院, 浙江 杭州 310036)
Foundation item:Supported by the Natural Science Foundation of Zhejiang Province(LY17A010018).
CHEN Huanyin(1963—), male, Professor, Ph.D., majored in algebra of basic mathematics.E-mail:huan- yinchen@aliyun.com
10.3969/j.issn.1674-232X.2016.06.012
O153.3 MSC2010: 13F25; 16S34; 16U10 Article character: A
1674-232X(2016)06-0623-09
Received date:2016-02-06
