黄 春, 孙峪怀, 李 钊, 张 健

(1. 四川师范大学 数学与软件科学学院, 四川 成都 610066; 2. 四川职业技术学院 应用数学与经济系, 四川 遂宁 629000)

(2+1)维非线性分数阶Zoomeron方程的新精确解

黄 春1,2, 孙峪怀1*, 李 钊1, 张 健1

(1. 四川师范大学 数学与软件科学学院, 四川 成都 610066; 2. 四川职业技术学院 应用数学与经济系, 四川 遂宁 629000)

通过复变换将高维非线性分数阶偏微分方程转化为整数阶常微分方程,然后利用扩展的(G′/G)-展开法,构建(2+1)维非线性分数阶Zoomeron方程的新精确解,其中包括含参数的双曲函数解、三角函数解和有理数解.

(2+1)维非线性分数阶Zoomeron方程; 扩展的(G′/G)-展开法; 精确解

1 预备知识

非线性分数阶偏微分方程是整数阶偏微分方程的推广,它比整数阶偏微分方程更全面地解释实际现象,并且能够深刻描述与反映物体内在的性质.非线性分数阶偏微分方程在流体力学、材料力学、生物学、等离子体物理学、金融学、化学等许多领域有着广泛的应用,因此研究非线性分数阶偏微分方程的性质以及解的情况具有重要的意义.

(2+1)维非线性分数阶Zoomeron方程[1-5]

(1)

修正的Riemann-Liouville分数阶导数

(2)

修正的Riemann-Liouville分数阶导数的性质:

(3)

(4)

(5)

(2+1)维非线性分数阶Zoomeron方程,最早由F.Calogero等[1]提出:当α=1,方程(1)是一个隐式非线性演化方程;文献[2]通过分数首次积分法获得分数阶Zoomeron方程的一些精确解;文献[3-5]分别用指数函数展开法、(G′/G)-展开法、首次积分法获得该方程在整数阶情形下的精确解.

最近,LiZ.B.等[7]提出如(7)式所表达的复变换将分数阶偏微分方程转化为整数阶常微分方程,该变换被广泛运用在非线性分数阶偏微分方程的求解中.构建非线性分数阶偏微分方程精确解的方法主要包括:分数指数函数展开法[8-10]、分数首次积分法[11-13]、分数Riccati映射法[14-15]、分数(G′/G)-展开法[16-18]等.本文通过作复变换将高维非线性分数阶偏微分方程转化为整数阶常微分方程,然后运用扩展的(G′/G)-展开法构建(2+1)维非线性分数阶Zoomeron方程的新精确解.

2 方法描述

考虑下面的非线性分数阶偏微分方程

(6)

其中,u=u(x,y,t)是未知函数,P是u及u的关于x、y、t各阶偏导数的多项式.

步骤 1 通过作复变换

(7)

其中,l和c为任意常数,且ω≠0.

将方程(6)转化为只含变量ξ的整数阶常微分方程

(8)

步骤 2 设方程(8)的解为(G′/G)多项式形式

(9)

其中,G=G(ξ)满足辅助方程

(10)

λ和μ为常数.正整数N可以通过平衡(8)式中最高阶导数项和非线性项确定.

步骤 3 将方程(9)代入(8)式,并利用方程(10)合并(G′/G)的相同幂次项,然后令(G′/G)的各次幂系数为零.

步骤 4 求解上述以ai(i=-N,…,0,…,N)为未知量的代数方程组,借助于Maple软件,从而获得方程(6)的精确解.

下面将运用此方法来构建(2+1)维非线性分数阶Zoomeron方程的新精确解.

3 运用与结果

首先对方程(1)作变换

得

(11)

将方程(11)左右两边同时积分2次,取第二次积分常数为零,可得

(12)

其中r为非零积分常数.设方程(12)有如下形式的解

(13)

通过平衡方程(12)中的u3和u″,则有3N=N+2,有N=1,方程(13)化为

(14)

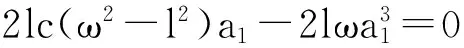
(15)

求解这个方程组可得:

(16)

(17)

情形 1 当λ2-4μ>0时,方程(1)有如下形式的双曲函数解:

特别地,当C1=0,C2≠0时有

(19)

当C1≠0,C2=0时有

(20)

(21)

其中

C1和C2为任意常数.

情形 2 当λ2-4μ<0时,方程(1)有如下形式的三角函数解:

特别地,当C1=0,C2≠0时有

(23)

当C1≠0,C2=0时有

(24)

(25)

其中

C1和C2为任意常数.

情形 3 当λ2-4μ=0时,方程(1)有如下形式的有理函数解:

(26)

(27)

其中

C1和C2为任意常数.

4 结果与讨论

本文通过作(7)式所表达的复变换将高维非线性分数阶偏微分方程转化为整数阶常微分方程,然后利用扩展的(G′/G)-展开法构建(2+1)维非线性分数阶Zoomeron方程的新精确解,其中包括含参数的双曲函数解、三角函数解和有理数解.文中所得结果u12(ξ)和u11(ξ)与文献[2]中的u1(ξ)和u2(ξ)一致,u32(ξ)和u31(ξ)与文献[2]中的u4(ξ)和u5(ξ)一致,u2、u4和u6是文献中未曾出现的结果.本文构建了分数阶Zoomeron方程的新精确解,同时也说明扩展的(G′/G)-展开法是求解一类非线性分数阶偏微分方程行之有效的方法.

[1] CALOGERO F, DEGASPERIS A. Nonlinear evolution equations solvable by the inverse spectral transform[J]. Il Nuovo Cimento,1976,B2(32):201-242.

[2] ZAYE E M E, AMER Y A. The first integral method and its application for finding the exact solutions of nonlinear fractional partial differential equations in the mathematical physics[J]. Inter J Phys Sci,2014,8(9):174-183.

[3] KHAN K, AKBAR M A. Traveling wave solutions of the (2+1)-dimensional Zoomeron equation and the Burgers equations via the MSE method and the exp-function method[J]. Ain Shams Engineering J,2014,5(1):247-256.

[5] ABAZARI R. The solitary wave solutions of Zoomeron equation[J]. Appl Math Sci,2011,59(5):2943-2949.

[5] BEKIR A, TASCAN F, ÜNSAL Ö. Exact solutions of the Zoomeron and Klein-Gordon-Zakharov equations[J]. J Association Arab Univ Basic Appl Sci,2015,17:1-5.

[6] JUMARIE G. Modified Riemann-Liouville derivative and fractional Taylor series of nondifferentiable functions further results[J]. Comput Math Appl,2006,51(9):1367-1376.

[7] LI Z B, HE J H. Fractional complex transform for fractional differential equations[J]. Appl Math Comput,2010,15(5):970-973.

[8] GÜNER Ö, BEKIR A, PANDIR Y. Solving nonlinear space-time fractional differential equations using the exp-function method[J]. AIP Conf Proc,2015,1648(1):1-5.

[9] YAN L M, XU F S. Generalized exp-function method for nonlinear space-time fractional differential equations[J]. Thermal Sci,2014,18(5):1573-1576.

[10] JIA S M, HU M S, CHEN Q L, et al. Exact solution of fractional Nizhnik-Novikov-Veselov equation[J]. Thermal Sci,2014,18(5):1716-1717.

[11] AMINIKHAH H, SHEIKHANI A R, REZAZADEH H. Exact solutions for the fractional differential equations by using the first integral method[J]. Nonlinear Engineering,2015,4(1):15-22.

[12] LU B. The first integral method for some time fractional differential equations[J]. J Math Anal Appl,2012,395(2):684-693.

[13] ESLAMI M, VAJARGAH F B, MIRZAZADEH M, et al. Application of first integral method to fractional partial differential equations[J]. Indian J Phys,2014,88(2):177-184.

[14] FENG Q H. A new fractional projective riccati equation method for solving fractional partial differential equations[J]. Commun Theory Phys,2014,62(2):167-172.

[15] ZHANG Y F, FENG Q H. Fractional riccati equation rational expansion method for fractional differential equations[J]. Appl Math Inform Sci,2013,7(4):1575-1584.

[16] ZHANG Y W. Solving STO and KD equations with modified Riemann-Liouville derivative using improved (G′/G)-expansion function method[J]. IAENG Inter J Appl Math,2015,45(1):16-22.

[17] ZHENG B. (G′/G)-expansion method for solving fractional partial differential equations in the theory of mathematical physics[J]. Commun Theory Phys,2012,58(5):623-630.

[18] YOUNIS M, ZAFAR A. Exact solution to nonlinear differential equations of fractional order via (G′/G)-expansion method[J]. Appl Math,2013,5(1):1-6.

2010 MSC：35K05

(编辑 李德华)

Received date:2015-02-03

Foundation Items:This work is supported by National Natural Science Foundation of China(No.11371267 and 11571245) and Basic Project of Sichuan Provincial Science and Technology Department(No.2016JY0204)

Construction of the New Exact Solutions for the (2+1) Dimensional Nonlinear Fractional Zoomeron Equation

HUANG Chun1,2, SUN Yuhuai1, LI Zhao1, ZHANG Jian1

( 1.CollegeofMathematicsandSoftwareScience,SichuanNormalUniversity,Chengdu610066,Sichuan;2.DepartmentofAppliedMathematicsandEconomy,SichuanVocationalandTechnicalCollege,Suining629000,Sichuan)

(2+1) dimensional nonlinear fractional Zoomeron equation has been discussed. Firstly, the (2+1) dimensional nonlinear fractional Zoomeron equation has been converted to a nonlinear ordinary differential equation by using the fractional complex transformation. Then, the extended (G′/G)-expansion method is used to construct exact solutions. A series new explicit solutions are obtained, which include hyperbolic function solutions, trigonometric function solutions and rational solutions, more results than existing ones.

(2+1) dimensional nonlinear fractional Zoomeron equation; extended (G′/G)-expansion method; exact solutions

2015-07-08

国家自然科学基金(11371267)和四川省教育厅自然科学重点基金(2012ZA135)

O175.29

A

1001-8395(2017)01-0051-04

10.3969/j.issn.1001-8395.2017.01.008

*通信作者简介：孙峪怀(1963—),男,教授,主要从事数学物理的研究,E-mail:sunyuhuai63@163.com