王忠民， 邹德志

(西安理工大学 土木建筑工程学院，西安　710048)



面内平动功能梯度斜板的主动振动控制

王忠民， 邹德志

(西安理工大学 土木建筑工程学院，西安710048)

对新型功能梯度材料制成的面内平动斜板，通过直角坐标系和斜角坐标系的坐标变换，建立了在斜角坐标系下受多个集中控制力作用的横向振动控制微分方程。采用微分求积法，将微分方程和边界条件对空间坐标进行离散化处理，得到了时域内振动控制系统的状态方程。应用最优控制法，对面内平动功能梯度斜板的无量纲运动速度小于一阶无量纲临界速度时的等幅振动和大于一阶无量纲临界速度时的发散失稳两种情况进行了数值仿真，得到控制前后若干个节点挠度随时间的变化曲线。结果表明，该方法能够有效地控制面内平动功能梯度斜板的横向振动，特别是对于发散失稳的抑制。

面内平动斜板；功能梯度材料；最优振动控制；微分求积法

在造船工业和桥梁工程等领域，斜板、加肋斜板的应用越来越广泛。目前，许多学者在面内平动(或轴向运动)矩形板或薄膜的线性振动、非线性振动、稳定性以及振动控制方面做了大量的研究。Gorman[1]用解析法研究了矩形板的自由振动特性。Lin[2]以对边简支对边自由的轴向运动板为对象，分析了轴向运动速度、长宽比和刚度对板的稳定性的影响。Shin等[3]分析了轴向运动矩形薄膜的振动特性。周银锋等[4]研究了轴向运动Kelvin-Voigt黏弹性矩形板的横向振动特性。阮苗等[5]分析了受切向均布随从力的功能梯度(Functionally Graded Materials,FGM)斜板的稳定性。Hossain Nezhad Shirazi等[6]利用模糊控制策略对功能梯度矩形板进行了控制，其中没有考虑板的轴向运动。贺容波等[7]提出了基于光电层合简支板的最优模糊多模态主动振动控制算法。浦玉学等[8]提出基于次级通道在线辨识的变步长振动主动控制算法，以某实时控制器进行了简支梁振动主动控制试验。在斜板结构的弯曲、屈曲及后屈曲研究方面，李国豪[9]提出了各向异性斜板弯曲的平衡微分方程及其实用的近似解法。纪冬梅等[10]采用Galerkin法，以小波作为试函数，得出了斜板在不同边长比与不同斜角下的后屈曲四级渐近解。黎振源等[11]分析了两对边简支、另两对边自由简支斜板桥振动频率特性，以及单辆标准车靠边行驶、靠中行驶和两辆标准车并排行驶时的车桥振动特性。阮苗等[12]研究了功能梯度斜板在两对边受有均布压力作用下的屈曲问题，讨论了斜板的几何外形尺寸、夹角、梯度指标以及中面变形等因素对临界屈曲荷载的影响。

在上述研究的基础上，对新型功能梯度材料制成的面内平动斜板，采用二元Diracδ函数，建立了在集中控制力作用下的横向振动控制微分方程；采用微分求积法，将微分方程和边界条件进行离散化处理，得到了振动控制系统的状态方程；应用最优控制法，确定了最优控制率，对系统进行数值仿真，得到了控制前后所求点的挠度随时间的变化曲线。

1　运动功能梯度斜板的控制微分方程

1.1受控系统的力学模型

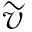
X=XcVc+XmVm=XcVc+Xm(1-Vc)

(1)

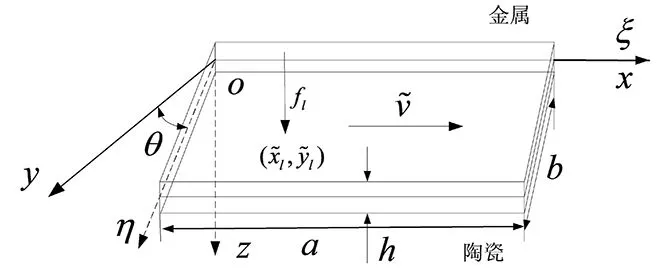
图1　面内平动的FGM斜板Fig.1 In-plane translating skew plate made of functionally graded materials

1. 2控制微分方程

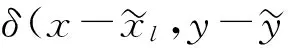
(2)

式中：w(x,y,t)是板的挠度函数；μ是泊松比，Em、ρm和Ec、ρc分别是金属、陶瓷的杨氏模量和密度，Ecm=Ec-Em，ρcm=ρc-ρm；4为重调和算子，即

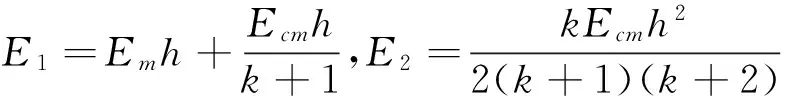
由图2知，直角坐标和斜角坐标的关系为

x=ξ+ηcosθ，y=ηsinθ

(3)

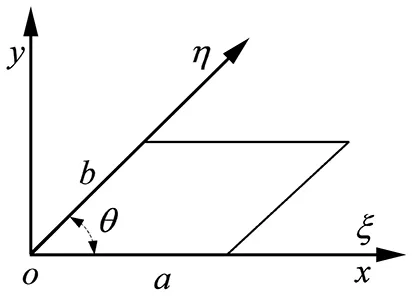
图2　直角坐标与斜角坐标Fig.2 Rectangular coordinates and skew coordinates

对控制微分方程(2)，应用式(3)，可得到斜角坐标系下的控制微分方程，即

(4)

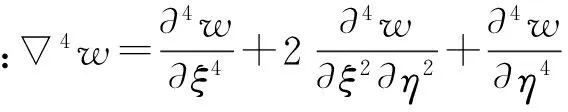
引入无量纲量

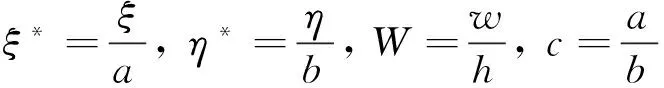
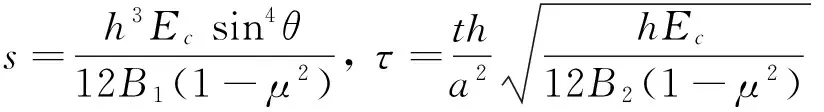
(5)

得到斜角坐标系下无量纲量表示的控制微分方程

(6)

1. 3微分方程的离散

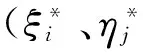
(7)

四边简支斜板的边界条件为

W1j=WNj=Wi1=WiN=0

(i,j=1,2,…,N)

(8a)

(i=2,N-1;j=2,3,…,N-1)

(8b)

(j=2,N-1;i=2,3,…,N-1)

(8c)

(9)

二元Diracδ(ξ*,η*)函数的展开式

(10)

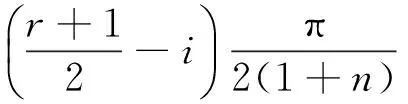
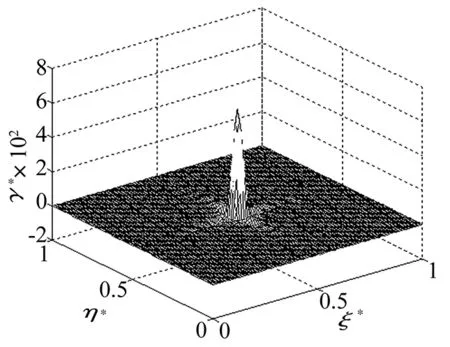
图3　二元Diracδ(ξ*-0.5,η*-0.5)函数的部分和随坐标变量的变化曲面Fig.3 Curved surface of partial sum for Dirac function δ(ξ*-0.5,η*-0.5) versus two variables

本文采用不均匀分布节点方式，即

(12)

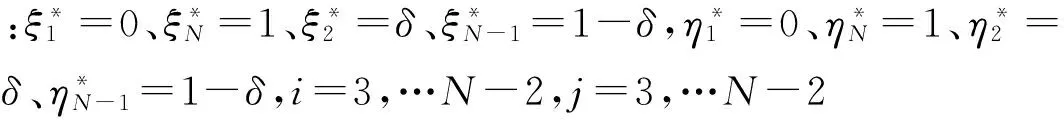
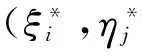
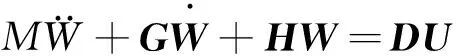
(13)

式中：U(τ)=[u1(τ),u2(τ),u3(τ),u4(τ),u5(τ)]T为无量纲控制力列阵。

2　最优控制器设计

将方程(13)写成状态方程

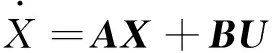
(14a)

Y=CX

(14b)

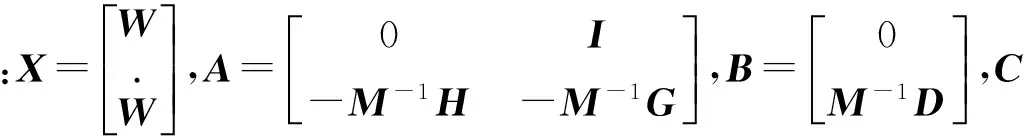
对于无限时间输出调节器，二次型指标为

(15)

用极小值原理[17]，得到唯一最优控制率

U*(τ)=-R-1BTPX(τ)

(16)

式中：P是正定对称常值矩阵，是下列Riccati代数方程的唯一解

PA+ATP-PBR-1BTP+Q=0

(17)

最优状态X*(τ)是下列微分方程和初始条件的解

X(τ0)=X0

(18)

在式(16)中，令K=-R-1BTP，有最优指标

(19)

受控系统闭环控制框图如图4所示。

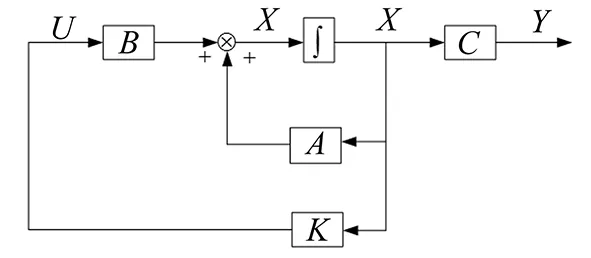
图4　受控系统闭环结构图Fig.4 Closed-loop structurediagram of the controlled system

3　数值算例

取板的边长a=b=1，即长宽比c=a/b=1；采用的FGM 板由 金属Aluminum 和陶瓷Zirconia两种材料构成，其弹性模量分别为Em=70 GPa和Ec=151 GPa，密度分别为ρm=2 707 kg/m3和ρc=3 000 kg/m3，泊松比μ=μm=μc=0.3；梯度指标k=1，斜板的夹角θ=75°，N=9。

对于无控制力作用的运动功能梯度斜板，前三阶复频率ω1、ω2、ω3的实部和虚部随无量纲速度v的变化曲线如图5所示。可以看出，当无量纲速度小于一阶无量纲临界速度5.49时，第一阶复频率的虚部是0，板是稳定的；当无量纲速度大于5.49小于6.9时，第一阶复频率虚部不为0，实部为0，斜板发生发散失稳。

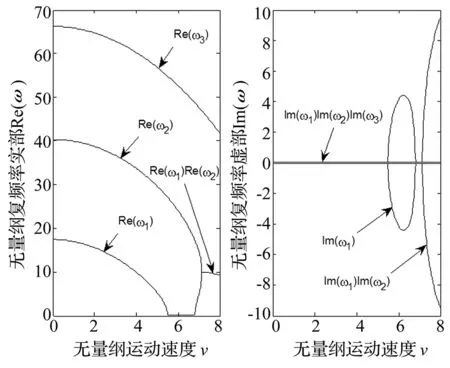
图5　前三阶无量纲复频率的实部与虚部随无量纲速度的变化曲线Fig.5 Curve of real part and imaginary part of the first three dimensionless complex frequency versus velocity

在以下分析中，取斜板上A1、A2、A3点为控制对象。为了清晰地表示这三个点的位置，将板画成矩形，如图6所示。在方程(14a)中，将B取成0矩阵，即无控制状态，取初始条件为

[000000000000000000

00000000000.10000000

-0.10000000000000]T

对无量纲运动速度v=3，用Runge-Kutta法计算了A1、A2、A3点处的无量纲挠度随无量纲时间的变化情况，如图7-图9(图中均为无量纲量)。由图可以看出，其变化规律是等幅稳态振动。

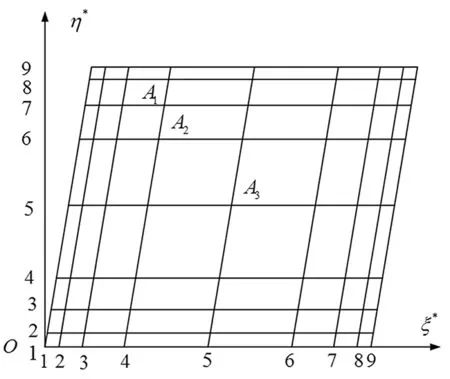
图6　A1、A2、A3点所在位置Fig.6 Location of point A1、A2、A3

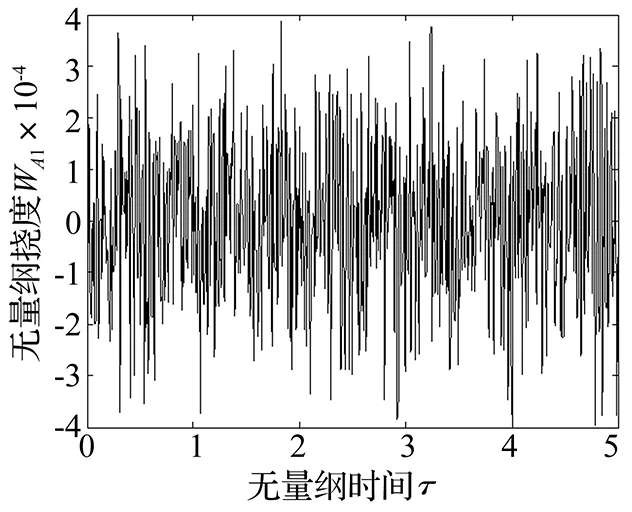
图7　控制前A1点挠度随时间的变化曲线(v=3)Fig.7 Curve for deflection of point A1 versus time under uncontrolled state(v=3)

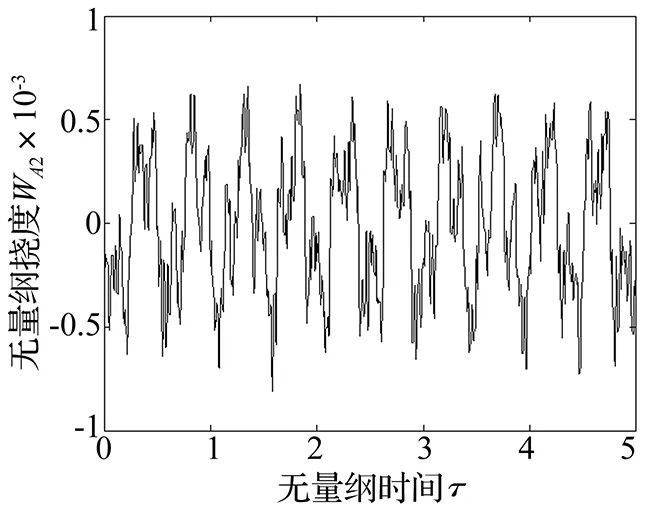
图8　控制前A2点挠度随时间的变化曲线图(v=3)Fig.8 Curve for deflection of point A2 versus time under uncontrolled state(v=3)

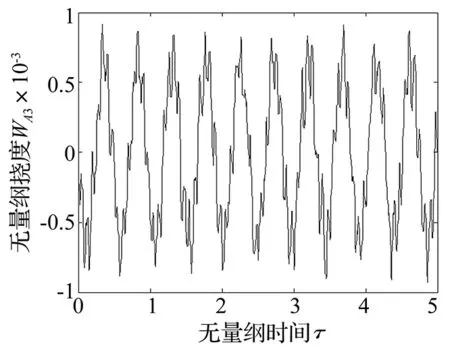
图9　控制前A3点挠度随时间的变化曲线(v=3)Fig.9 Curve for deflection of point A3 versus time under uncontrolled state(v=3)

D3=[0.000 028 109 162 15,0.000 083 491 291 43,

-0.013 192 615 874 43,0.000 083 491 291 43,

0.000 028 109 162 15,0.000 083 491 291 43,

0.000 247 990 164 49,-0.039 185 391 967 64,

0.000 247 990 164 49,0.000 083 491 291 43,

-0.013 192 615 874 43,-0.039 185 391 967 64,

6.191 757 430 466 36,-0.039 185 391 967 64,

-0.013 192 615 874 43,0.000 083 491 291 43,

0.000 247 990 164 49,-0.039 185 391 967 64,

0.000 247 990 164 49,0.000 083 491 291 43,

0.000 028 109 162 15,0.000 083 491 291 43,

-0.013 192 615 874 43,0.000 083 491 291 43,

0.000 028 109 162 15]T

对方程式(14a)和式(16)～(18)进行数值计算，得到控制后的无量纲挠度随无量纲时间的变化曲线如图10～图12所示(图中均为无量纲量)。从这些响应曲线可以看出，控制后板的无量纲挠度随无量纲时间的响应曲线呈现为显著的衰减，斜板的振动受明显的抑制，在无量纲时间大于2 s后，无量纲挠度几乎趋于0。

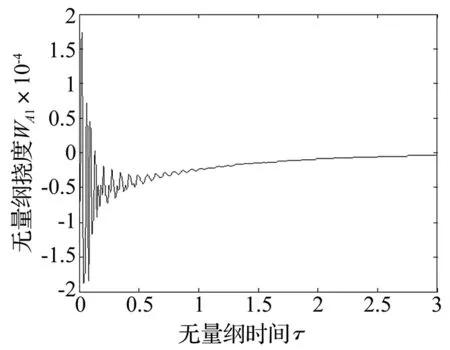
图10　控制后A1点挠度随时间的变化曲线(v=3)Fig.10 Curve for deflection of point A1versus time under controlled state(v=3)

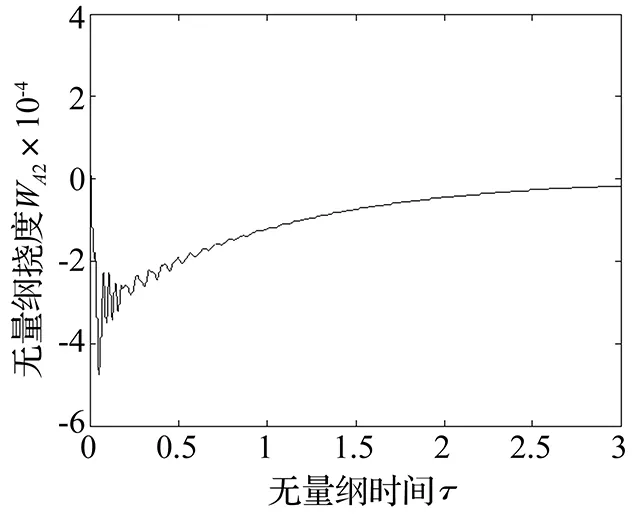
图11　控制后A2点挠度随时间的变化曲线(v=3)Fig.11 Curve for deflection of point A2versus time under controlled state(v=3)

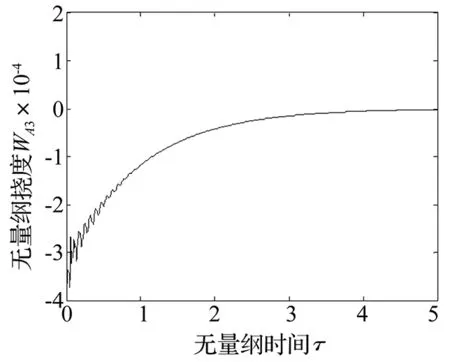
图12　控制后A3点挠度随时间的变化曲线(v=3)Fig.12 Curve for deflection of point A3 versus time under controlled state(v=3)

在图5中，当斜板的无量纲速度v=6时，斜板呈现为发散失稳状态，导致产生发散失稳的主要原因是第一阶复频率的虚部Im(ω1)=-4.136， 实部是0。v=6时的响应曲线如图13～图15，文中只画出前两秒的变化曲线，可以看出各点的挠度绝对值随时间的增加越来越大。实施控制后响应曲线如图16-图18所示，显然斜板的无量纲挠度绝对值的增大现象得到明显的抑制，使其在短时间内趋近于0，最优控制效果非常明显。在最优控制中，合理选择加权矩阵Q和R更利于确定最优控制律。

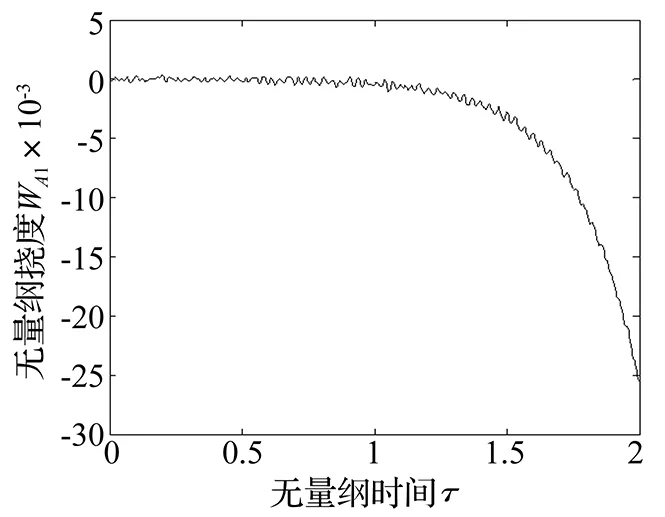
图13　控制前A1点挠度随时间的变化曲线(v=6)Fig.13 Curve for deflection of point A1 versus time under uncontrolled state(v=6)

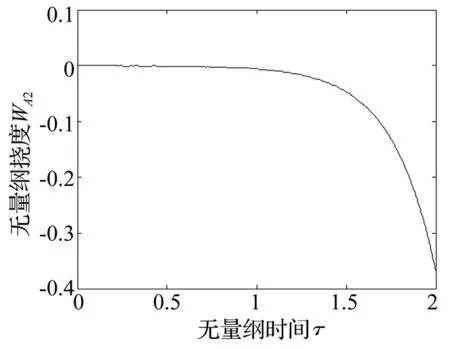
图14　控制前A2点挠度随时间的变化曲线(v=6)Fig.14 Curve for deflection of point A2 versus time under uncontrolled state(v=6)

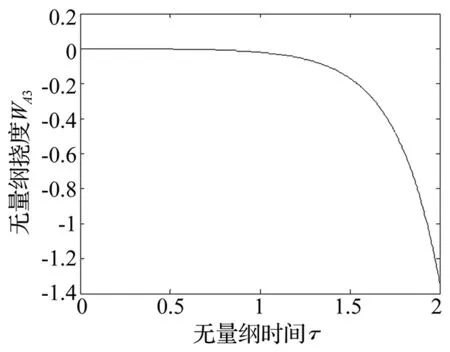
图15　控制前A3点挠度随时间的变化曲线(v=6)Fig.15 Curve for deflection of point A3versus time under uncontrolled state(v=6)

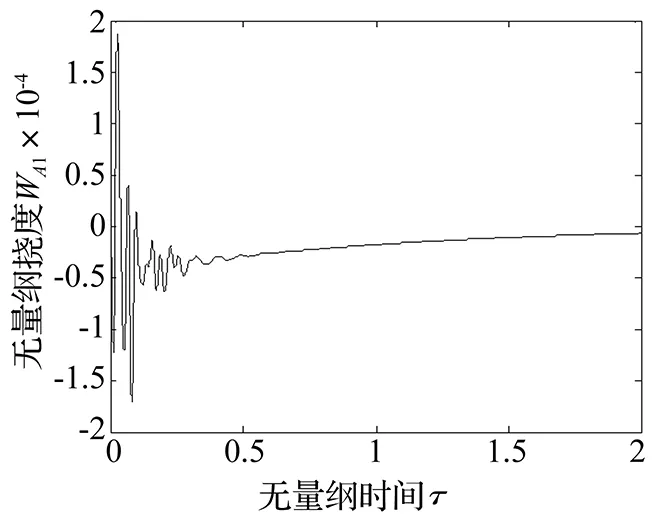
图16　控制后A1点挠度随时间的变化曲线(v=6)Fig.16 Curve for deflection of point A1 versus time under controlled state(v=6)

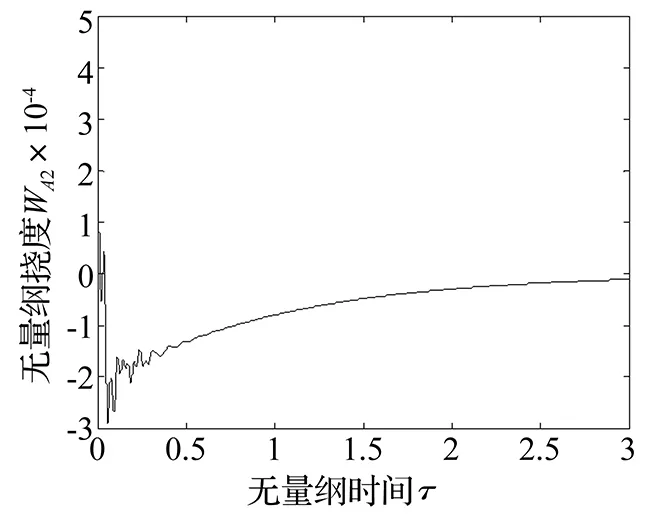
图17　控制后A2点挠度随时间的变化曲线(v=6)Fig.17 Curve for deflection of point A2versus time under controlled state(v=6)

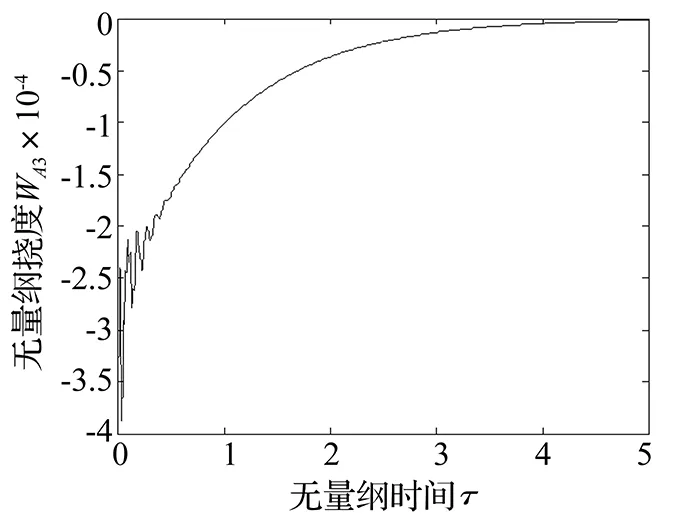
图18　控制后A3点挠度随时间的变化曲线(v=6)Fig.18 Curve for deflection of point A3versus time under controlled state(v=6)

4　结　论

利用微分求积法，对面内平动功能梯度斜板的控制微分方程进行了空间离散，建立了时域内的状态方程。应用Runge-Kutta法，对无控制的斜板，计算了面内平动速度小于一阶无量纲临界速度(v=3，等幅振动)和大于一阶临界速度(v=6，发散失稳)两种情况的斜板挠度随时间的变化情况。再用最优控制理论，求得了最优控制率，并对斜板在这两种情况下稳态振动和发散失稳分别进行了主动控制。仿真结果表明了该法的可行性和有效性。

[1] Gorman D J. Free vibration analysis of rectangular plates[M]. New York: Elsevier North Holland, 1982.

[2] Lin C C. Stability and vibration characteristics of axially moving plates[J]. International Journal of Solids and Structures, 1997, 34(24): 3179-3190.

[3] Changho S, Jintai C, Wonsuk K. Dynamic characteristics of the out-of-plane vibration for an axially moving membrane [J]. Journal of Sound and Vibration, 2005, 286(4/5): 1019-1031.

[4] Zhou Yin-feng, Wang Zhong-min. Transverse vibration characteristics of axially moving viscoelastic plate[J]. Applied Mathematics and Mechanics, 2007, 28(2): 209-218.

[5] Ruan Miao, Wang Zhong-min, Wang Yan. Dynamic stability of functionally graded materials skew plates subjected to uniformly distributed tangential follower forces[J]. Journal of Vibration and Control, 2012, 18(7): 913-923.

[6] Hossain Nezhad Shirazi A, Qwji H R, Rafeeyan M. Active vibration control of an FGM rectangular plate using fuzzy logic controllers[C]//Procedia Engineering,2011,14:3019-3026.

[7] 贺容波, 郑世杰. 光电层合简支板的多模态最优模糊主动振动控制[J]. 振动与冲击，2015, 34(10): 77-81.

HE Rong-bo，ZHENG Shi-jie. Multi-modal optimal fuzzy active vibration control of a photo-electric laminated simplysupported plate[J]. Journal of Vibration and Shock, 2015, 34(10): 77-81.

[8] 浦玉学，张方，姜金辉. 变步长自适应结构振动主动控制算法[J]. 振动与冲击，2015, 34(8):199-205.

PU Yu-xue，ZHANG Fang，JIANG Jin-hui. A varying step adaptive algorithm for structural vibration active control[J]. Journal of Vibration and Shock, 2015, 34(10): 199-205.

[9] 李国豪. 关于斜交异性斜板的弯曲理论[J]. 同济大学学报, 1997, 25(2): 121-126.

LI Guo-hao. On the bending theory of skew anisotropic skew plate[J]. Journal of Tongji University, 1997, 25(2): 121-126.

[10] 纪冬梅, 胡毓仁. 小波加权残值法在斜板后屈曲上的应用[J]. 应用力学学报, 2008, 25(4): 673-677.

JI Dong-mei, HU Yu-ren. Wavelet weighted residuals with application to post-buckling analysis of skew plates[J]. Chinese Journal of Applied Mechanics, 2008, 25(4): 673-677.

[11] 黎振源, 夏桂云, 李传习. 简支斜板的车-桥耦合振动分析[J]. 交通科学与工程, 2010, 26(1): 59-65.

LI Zhen-yuan, XIA Gui-yun, LI Chuan-xi. Vibrating frequencies and vehicle-bridge vibration of simply- supported skewed slab[J]. Journal of Transport Science and Engineering, 2010, 26(1): 59-65.

[12] 阮苗, 王忠民. 功能梯度斜板的屈曲分析[J]. 机械工程学报, 2011, 47(6):57-61.

RUAN Miao, WANG Zhong-min. Buckling analysis of functionally graded skew thin plate[J]. Journal of Mechanical Engineering, 2011, 47(6):57-61.

[13] Ruan Miao,Wang Zhong-min. Transverse vibrations of moving skew plates made of functionally graded material[J]. Journal of Vibration and Control, first published on December,2014,17:1-14.

[14] 王永亮. 微分求积法和微分求积单元法的原理与应用[D]. 南京: 南京航天航空大学, 2001.

[15] 何甲兴, 王淑云, 杨明. Fourier级数的求和理论与方法—求和因子法求和[J]. 数学的实践与认识, 2003, 33(12): 112-118.

HE Jia-xing, WANG Shu-yun, YANG Ming. On summation theory and method of Fourier series-summing by summation factor[J]. Mathematics in Practice and Theory,2003,33(12):112-118.

[16] 金钰. 二元傅里叶级数的收敛阶[J]. 宁夏师范学院学报:自然科学版, 2008, 29(6): 80-82.

JIN Yu. The convergent order of a double Fourier series[J]. Journal of Ningxia Teaches University:Natural Science, 2008, 29(6): 80-82.

[17] 关新平, 吴忠强. 现代控制理论[M]. 北京: 电子工业出版社, 2012.

Active vibration control for an in-plane translating skew plate made of functionally graded materials

WANG Zhongmin, ZOU Dezhi

(School of Civil Engineering and Architecture, Xi’an University of Technology, Xi’an 710048, China)

Through the coordinate transformation between an orthogonal coordinate system and a skew angle coordinate system, the transverse vibration control differential equation for an in-plane translating skew plate made of a new kind of functionally graded materials subjected to multiple concentrated control forces was derived in the skew angle coordinate system. The differential quadrature method was used to discretize the differential equation and boundary conditions, and the state equations of the vibration control system in time domain were built. Using the optimal control method, the optimal control law was obtained. For the cases that the axially dimensionless moving velocity of an in-plane translating skew plate was less than the first order dimensionless critical speed(equal amplitude vibration) and it was greater than the first order dimensionless critical speed(divergence and instability), some numerical simulations for the system were implemented, and varying curves of deflections of some certain nodes versus time under uncontrolled and controlled conditions were plotted. The numerical results showed that the optimal control method can effectively control the vibration of the in-plane translating skew plate made of functionally graded materials, particularly, suppress the divergence and instability of the plate.

in-plane translating skew plate; functionally graded materials; optimal vibration control; differential quadrature method

国家自然科学基金项目(11272254)；陕西省自然科学基础研究计划项目(2015JM1029)

2015-03-12修改稿收到日期：2015-08-05

王忠民 男，博士, 教授, 博士生导师，1957年生

O326

A

10.13465/j.cnki.jvs.2016.15.014