Ushangi GOGINAVA

Department of Mechanics and Mathematics，Tbilisi State University，Chavchavadze str.1，Tbilisi 0128，Georgia

E-mail∶zazagoginava@gmail.com

K´aroly NAGY

Institute of Mathematics and Computer Sciences，College of Ny´iregyh´aza，S´ost´oi str.31/B，4400 Ny´iregyh´aza，Hungary

E-mail∶nkaroly@nyf.hu



WEAK TYPE INEQUALITY FOR THE MAXIMAL OPERATOR OF WALSH-KACZMARZ-MARCINKIEWICZ MEANS∗

Ushangi GOGINAVA

Department of Mechanics and Mathematics，Tbilisi State University，Chavchavadze str.1，Tbilisi 0128，Georgia

E-mail∶zazagoginava@gmail.com

K´aroly NAGY

Institute of Mathematics and Computer Sciences，College of Ny´iregyh´aza，S´ost´oi str.31/B，4400 Ny´iregyh´aza，Hungary

E-mail∶nkaroly@nyf.hu

The main aim of this article is to prove that the maximal operator σκ∗of the Marcinkiewicz-Fej´er means of the two-dimensional Fourier series with respect to Walsh-Kaczmarz system is bounded from the Hardy space H2/3to the space weak-L2/3.

Walsh-Kaczmarz system，Marcinkiewicz-Fej´er means，Maximal operator，a.e. convergence，weak type inequality

2010 MR Subject Classification42C10

1　Introduction

For the two-dimensional Walsh-Fourier series，Weisz［1］proved that the maximal operator

is bounded from the two-dimensional dyadic martingale Hardy space Hpto the space Lpfor p＞2/3.The d-dimensional version of this result was reached in［2］.In［3］the author proved that in theorem of Weisz the assumption p＞2/3 is essential；in particular it was showed that the maximal operator σw∗is not bounded from the Hardy space H2/3to the space L2/3. On the other hand，it is proved that in the endpoint case p=2/3，the maximal operator of the Marcinkiewicz-Fej´er means of the double Walsh-Fourier series is bounded from the dyadic Hardy space H2/3to the space weak-L2/3［4］.

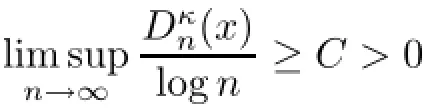
In 2006，the almost everywhere convergence of the Marcinkiewicz-Fej´er means of twodimensional Walsh-Kaczmarz-Fourier series was showed［15］.Moreover，the second author discussed the properties of the maximal operator，that is，the maximal operator is of weak type（1，1）and of type（p，p）for all 1＜p≤∞.In［16，17］，it was proven that the maximal operatoris bounded from the dyadic Hardy-Lorentz space Hpinto Lpspace for every p＞2/3. We also proved that the assumption p＞2/3 is essential［18］；in particular，we showed that the maximal operatoris not bounded from the Hardy space H2/3to the space L2/3.

In this article，we generalize the results of［15-17］for maximal operatorand prove that the maximal operatorof the Marcinkiewicz-Fej´er means with respect to the Walsh-Kaczmarz system is bounded from the Hardy space H2/3to the space weak-L2/3.

2　Definitions and Notations

Let P denote the set of positive integers，N：=P∪｛0｝.Denote Z2the discrete cyclic group of order 2，that is Z2=｛0，1｝，where the group operation is the modulo 2 addition and every subset is open.The Haar measure on Z2is given such that the measure of a singleton is 1/2.Let K be the complete direct product of the countable infinite copies of the compact groups Z2.The elements of K are of the form x=（x0，x1，···，xk，···）with coordinates xk∈｛0，1｝（k∈N）. The group operation on K is the coordinate-wise addition，and the measure（denoted byµ）and the topology are the product measure and topology.The compact Abelian group K is called the Walsh group.A base for the neighbourhoods of K can be given in the following way［5，19］：

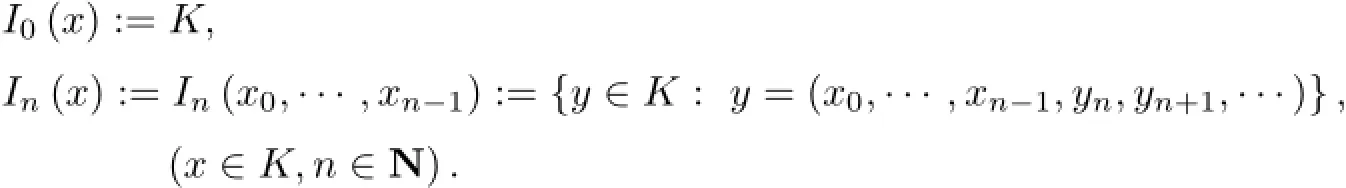
These sets are called dyadic intervals.Let 0=（0：i∈N）∈K denote the null element of K，In：=In（0）（n∈N）.Set en：=（0，···，0，1，0，···）∈K，the nth coordinate of which is 1and the rest are zeros（n∈N）.

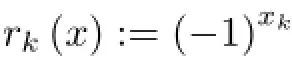
For k∈N and x∈K，denote the kth Rademacher function.can be written，where ni∈｛0，1｝（i∈N），that is，n is expressed in the number system of base 2.Denote|n|：=max｛j∈N：nj6=0｝，that is 2|n|≤n＜2|n|+1.

The Walsh-Paley system is defined as the sequence of Walsh-Paley functions：

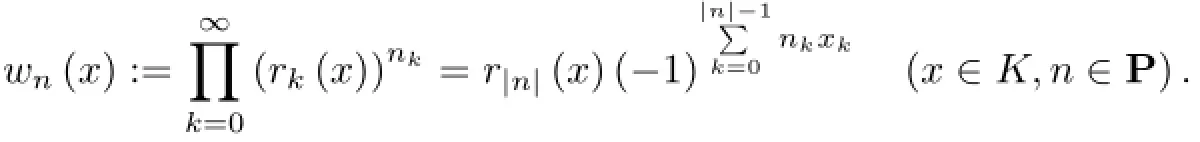
The Walsh-Kaczmarz functions are defined by κ0：=1 and for n≥1，

For A∈N，define the transformation τA：K→K by

By the definition of τA（see［9］），we have

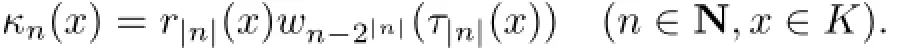
The norm（or quasinorm）of the space Lpis defined by

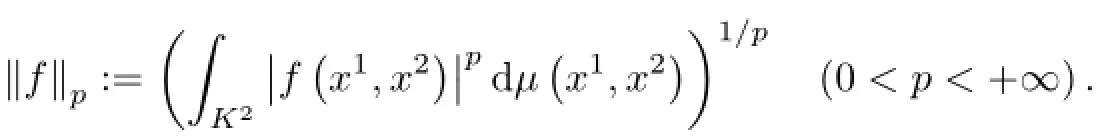
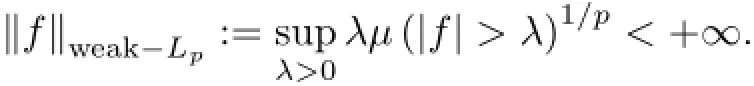
The σ-algebra generated by the dyadic 2-dimensional cubes Ik×Ikof measure 2−2kwill be denoted by Fk（k∈N）.

Denote by f=?f（n），n∈N?a one-parameter martingale with respect to（Fn，n∈N）（for details，see，for example，［1］）.The maximal function of a martingale f is defined by

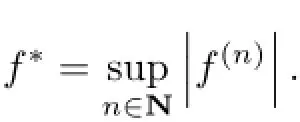
The space weak-Lpconsists of all measurable functions f for which

In case f∈L1，the maximal function can also be given by

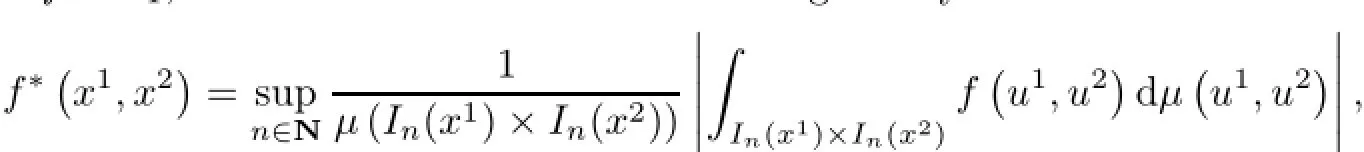
where?x1，x2?∈K2.

For 0＜p＜∞，the Hardy martingale space Hpconsists of all martingales for which

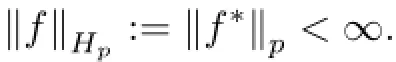
A bounded measurable function a is a p-atom if there exists a dyadic 2-dimensional cube I2，suchR that

a）I2adµ=0；

b）‖a‖∝≤µ（I2）−1/p；

c）supp a⊂I2.

An operator T which maps the set of martingale into the collection of measurable functions are called p-quasi-local，if there exists a constant Cp＞0 such that for every p-atom a，

holds，where I2is the support of the atom a.

The Dirichlet kernels are defined by

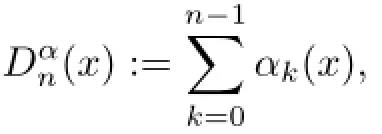
where αk=wk（for all k∈P）or κk（for all k∈P）.Recall that

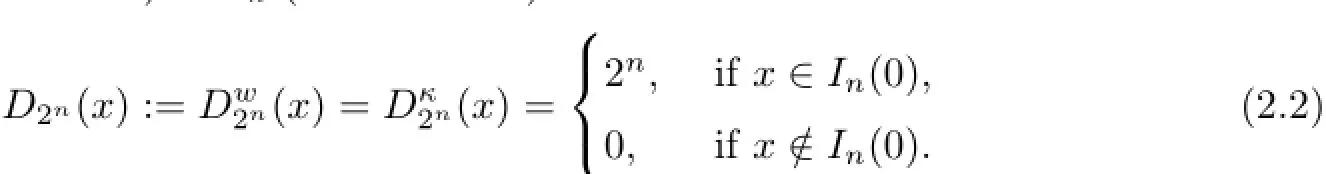
The Fej´er kernels are defined as follows：

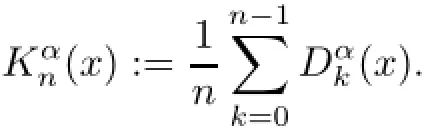
The Kronecker product（αn，m：n，m∈N）of two Walsh（-Kaczmarz）system is said to be the two-dimensional Walsh（-Kaczmarz）system.Thus，

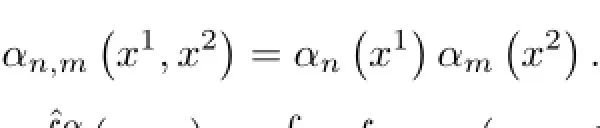
If f∈L1，then the numberˆfα（n，m）：=K2fαn，m（n，m∈N）is said to be the（n，m）th Walsh-（Kaczmarz-）Fourier coefficient of f.We can extend this definition to martingales in the usual way（see Weisz［1，20］）.Denote by Sαn，mthe（n，m）th partial sum of the Walsh-（Kaczmarz-）Fourier series of a martingale f.Namely，

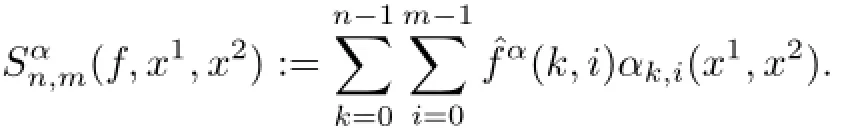
The Marcinkiewicz-Fej´er means of a martingale f are defined by

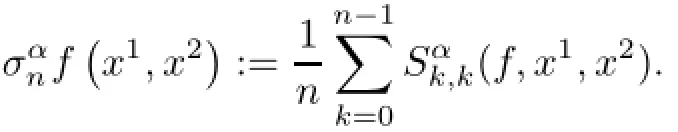
In 1939，Marcinkiewicz［21］has proved for f∈LlogL（［0，2π］2）that the means σnf defined for the two-dimensional trigonometric Fourier series converge a.e.to f as n→∞.Zhizhiashvili［22］improved this result for f∈L（［0，2π］2）.The analogous result for Walsh system belongs to Weisz［23］；for Vilenkin system belongs to G´at［24］.

The 2-dimensional Dirichlet kernels and Marcinkiewicz-Fej´er kernels are defined by

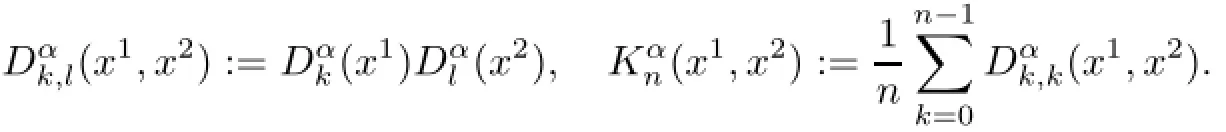
For the martingale f，we consider the maximal operators

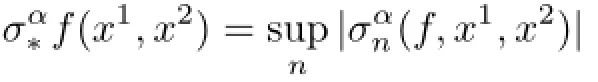
and

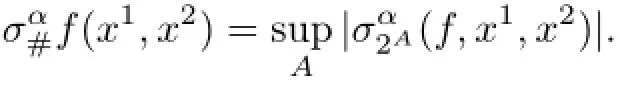
3　Formulation of Main Results

Theorem 3.1The maximal operatoris bounded from the Hardy space H2/3to the space weak-L2/3.

Corollary 3.2（G´at，Goginava and Nagy［17］）Let p＞2/3.Then the maximal operatoris bounded from the Hardy space Hpto the space Lp.

4　Auxiliary Propositions

We shall need the following lemmas（see［1，17］）.

Lemma 4.1（Weisz［1］）Suppose that an operator V is sublinear and，for some 0＜p＜1，

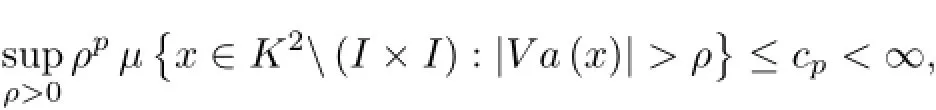
for every p−atom a，where I×I denotes the support of the atom.If V is bounded from Lp1to Lp1for a fixed 1＜p1≤∞，then

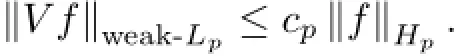
Lemma 4.2（G´at，Goginava and Nagy［17］）Let m1≤m2，A＞N，n＜2A+1，and

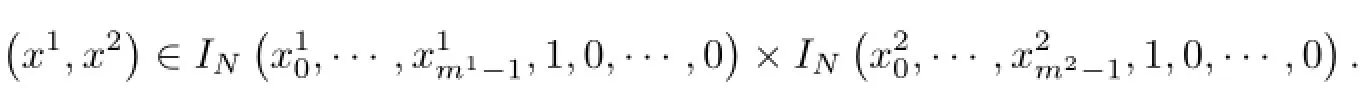
Then，

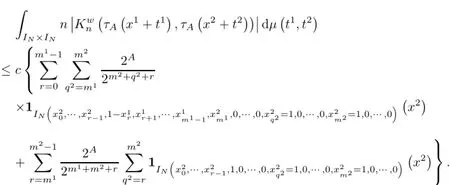
Lemma 4.3（G´at，Goginava and Nagy［17］）Let A＞N，n＜2A+1，and

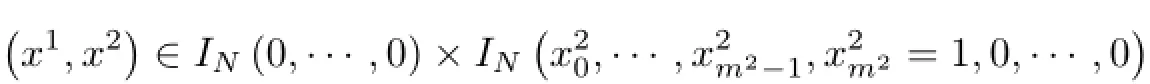
Then，

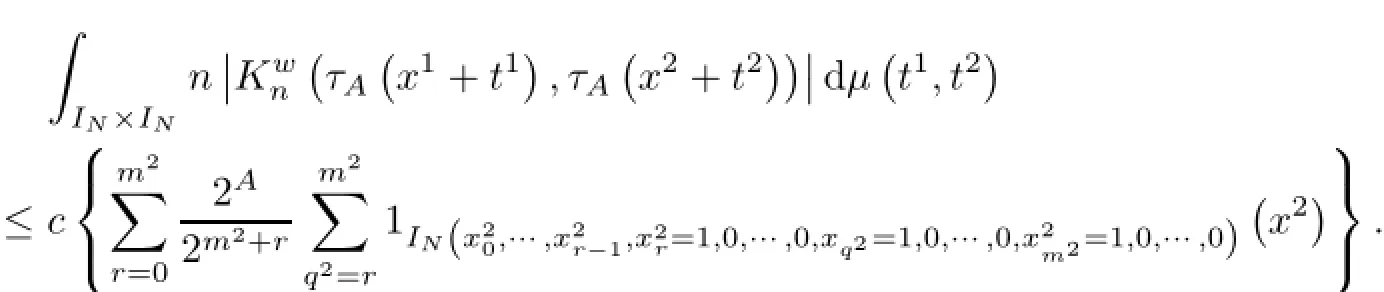
Lemma 4.4（G´at，Goginava and Nagy［17］）Let A＞N，n＜2A+1，and

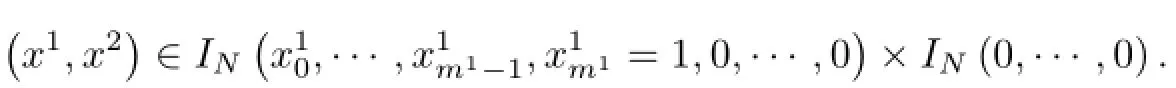
Then，

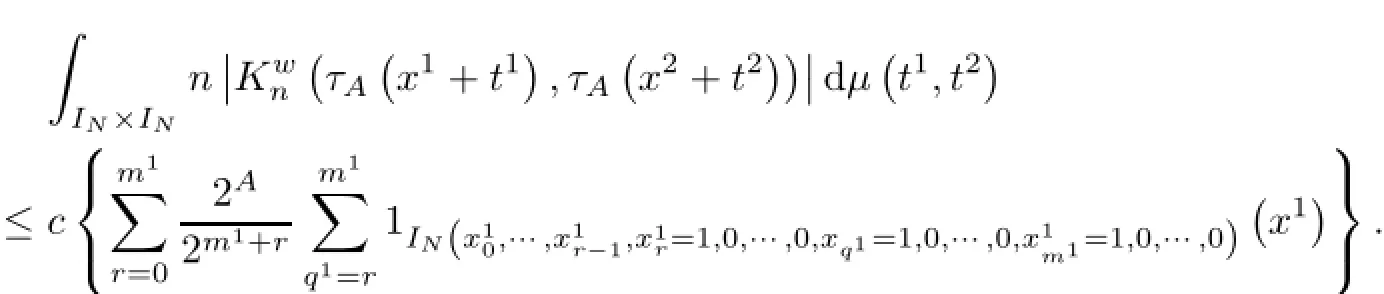
Lemma 4.5（Nagy［25］）For k∈P and（x1，x2）∈K2，we have

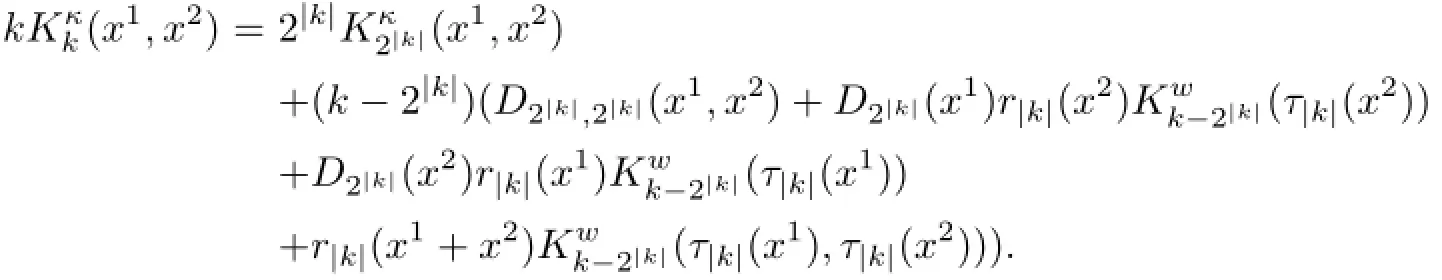
Lemma 4.6（G´at，Goginava and Nagy［16］）Let p＞1/2.Then the maximal operatoris p-quasi-local（see inequality（2.1））.

5　Proofs of Main Results

Proof of Theorem 3.1We apply Lemma 4.1，and suppose that a∈L∝is a 2/3-atom with support IN×IN.As σna?x1，x2?=0，if n≤2Nwe may assume that n＞2N（|n|≥N）.

It is evident that

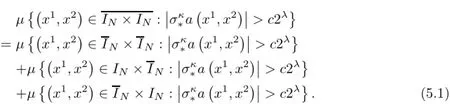
Step 1.Calculating over（KIN）×（KIN）.Let n=2A+m，where 0≤m＜2A（that is，|n|=A）.Then from Lemma 4.5 and by equality（2.2），we have

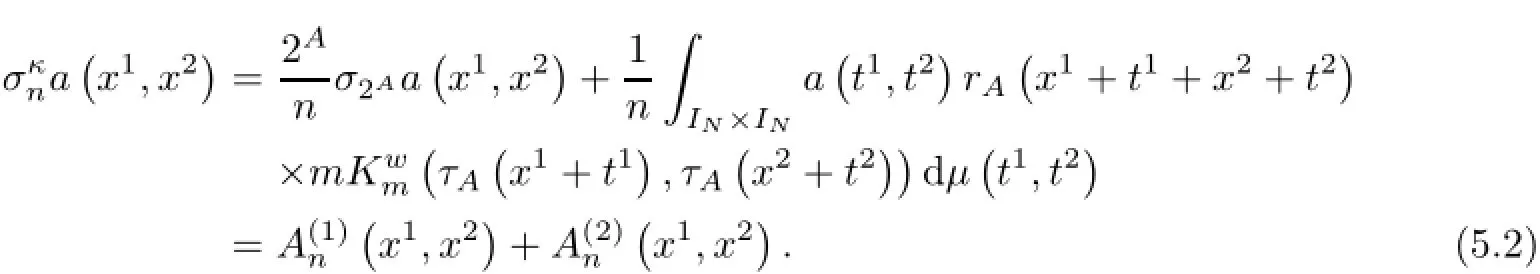
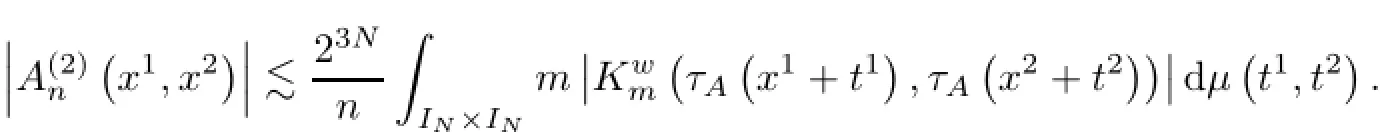
Let m1，m2=0，···，N−1 such that

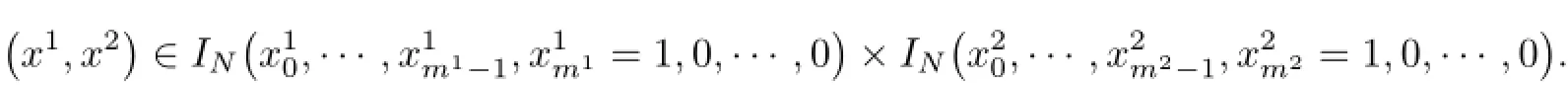
Then，applying Lemma 4.2，we get

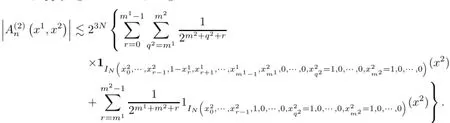
Set

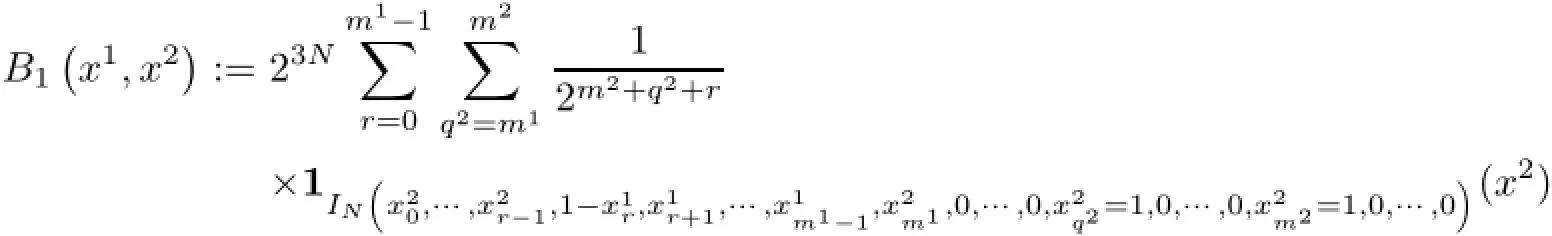
and

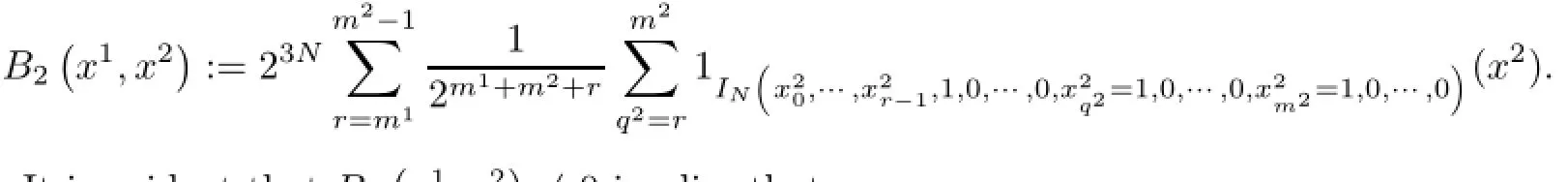
It is evident that B1x1，x26=0 implies that

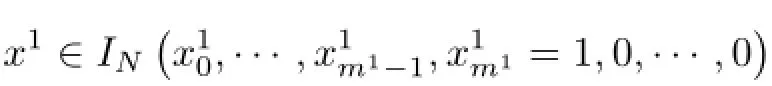
and

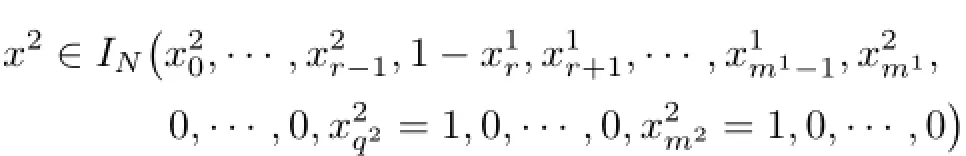
for some r and q2，where 0≤r＜m1，m1≤q2≤m2.

Consequently，

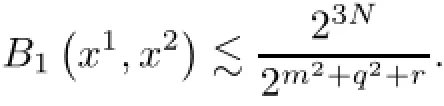
Let

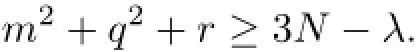
As

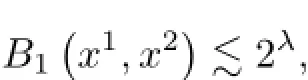
we obtain

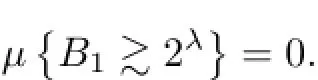
Hence，we can suppose that

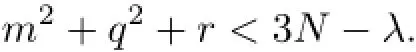
It is simple to calculate

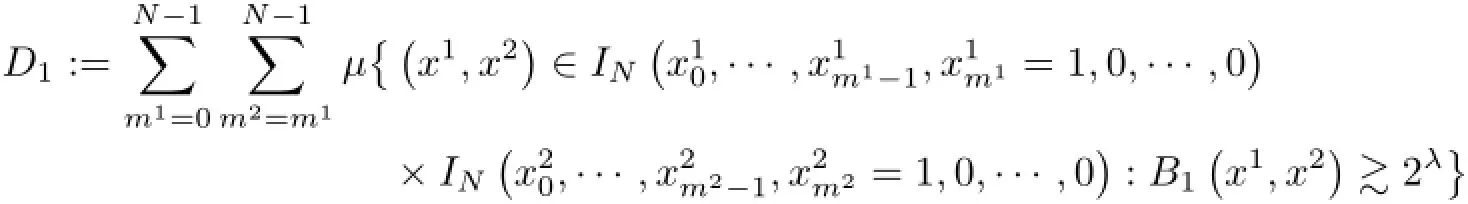
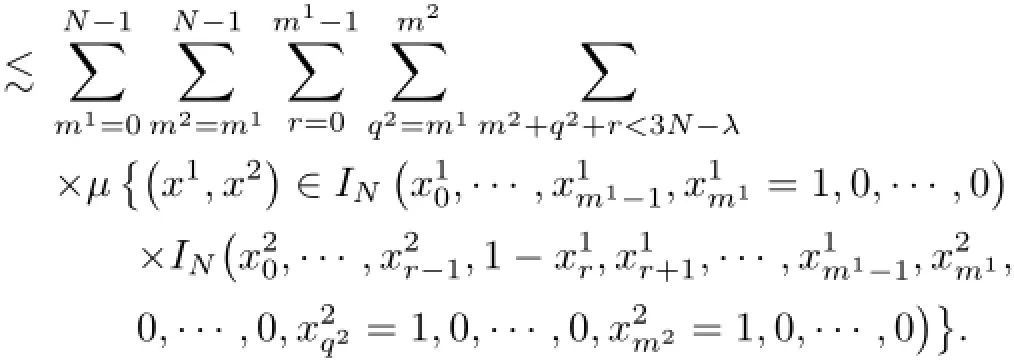
Set

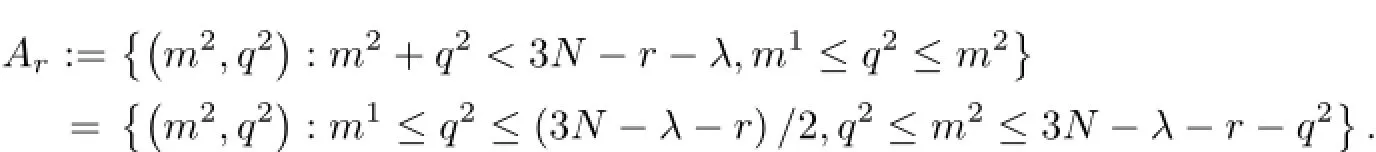
Let

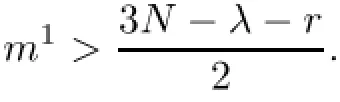
As

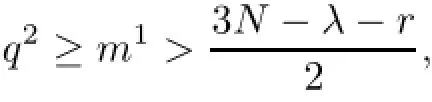
we conclude thatm2，q2/∈Ar.Hence，we can suppose that

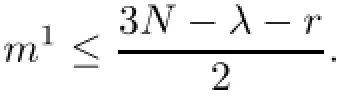
Then，it is evident that

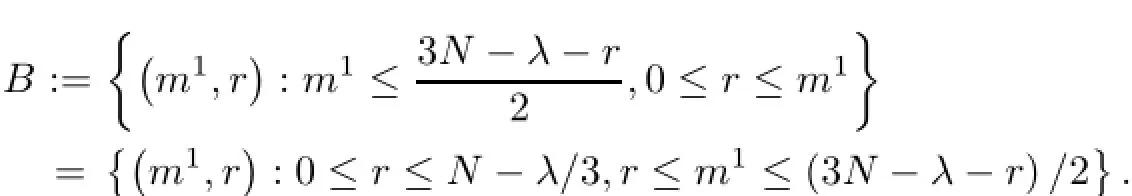
Consequently， Now，we discuss the term B2.It is evident that B2x1，x26=0 implies that

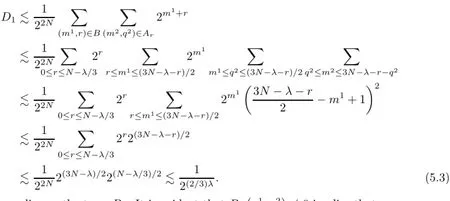
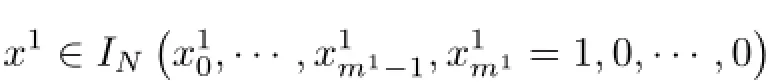
and

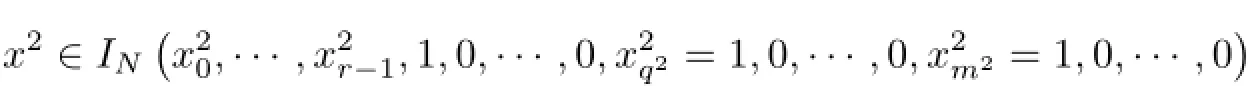
for some m1≤r≤m2，r≤q2≤m2.

Consequently，

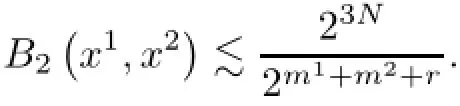
Let

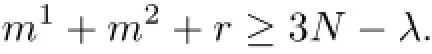
As

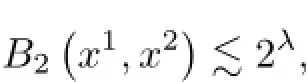
we conclude that

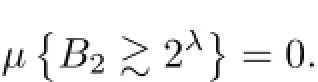
Hence，we can suppose that

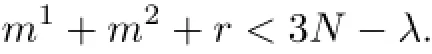
Set

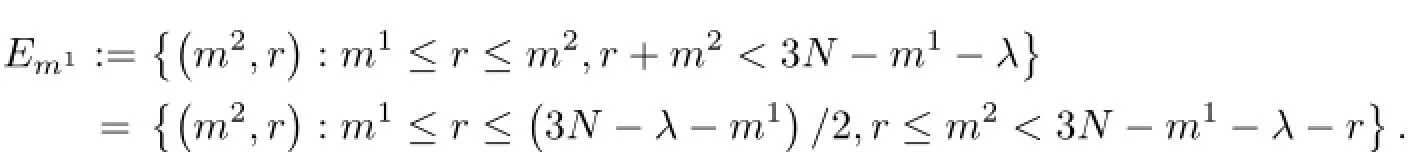
Then，we can write

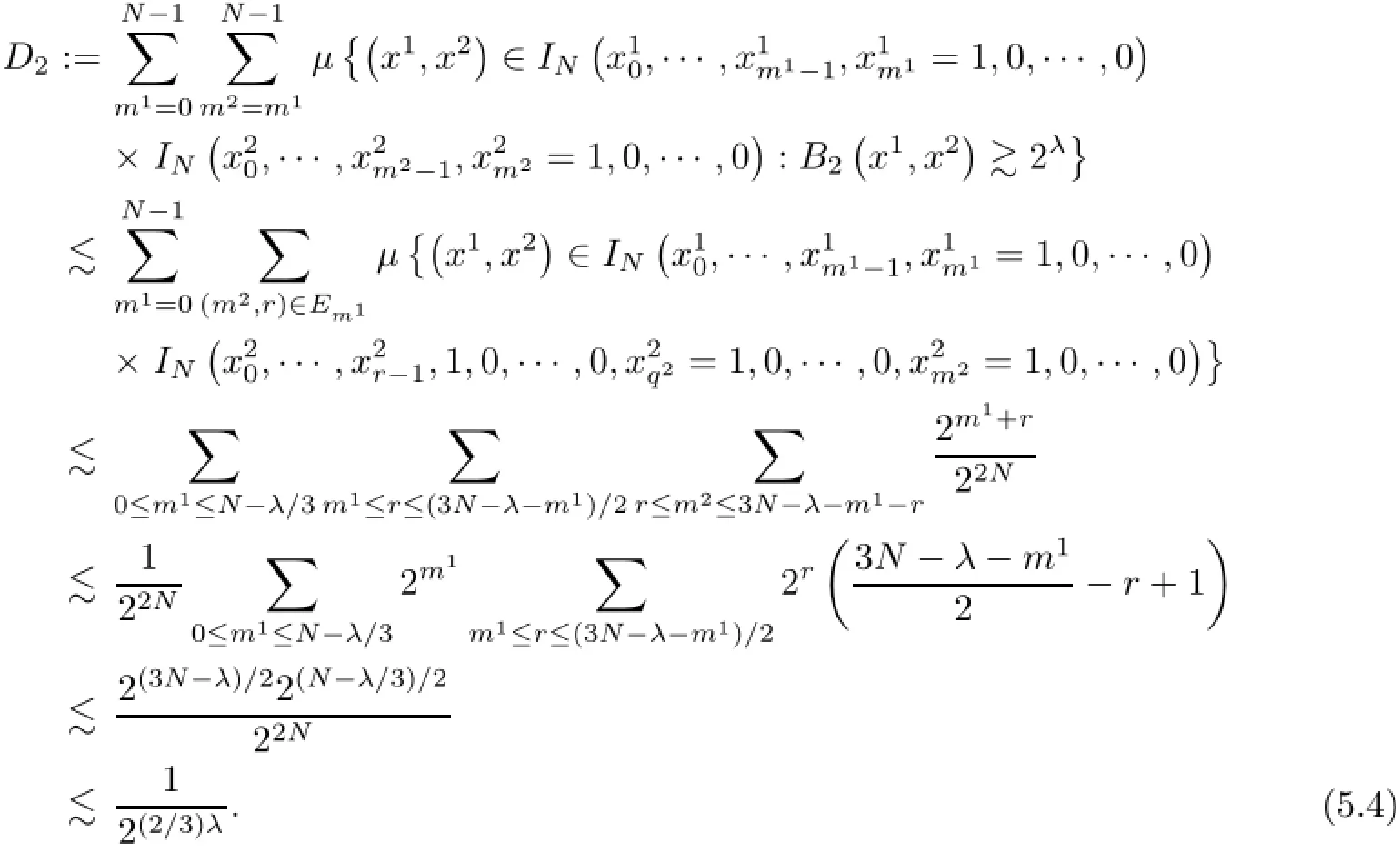
Combining（5.2）-（5.4），we conclude that

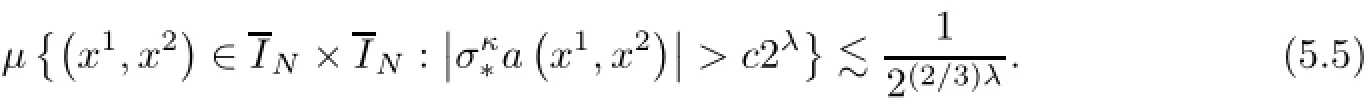
Step 2.Calculating over IN×（KIN）.By Lemma 4.5 and equality（2.2），we have

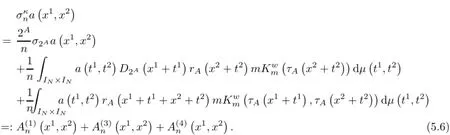
As（［17］）

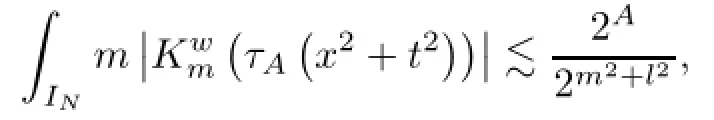
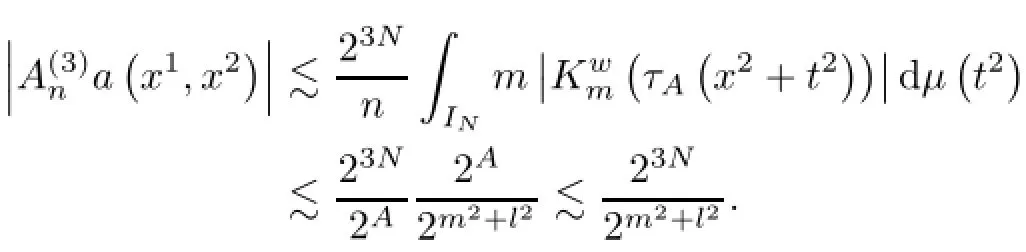
Then，

implies that

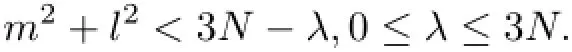
Then，we have

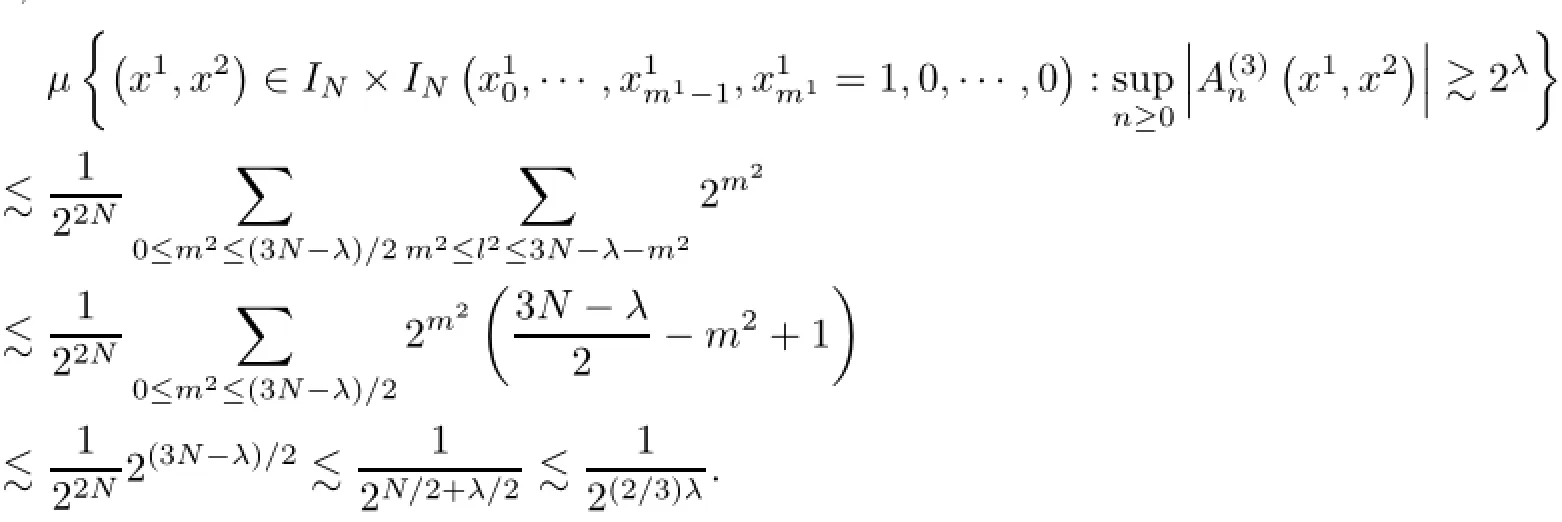
Applying Lemma 4.3，we get

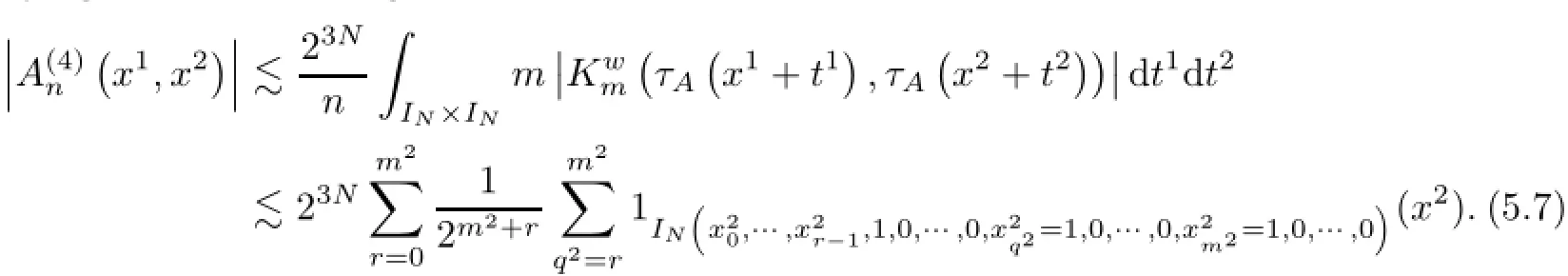
Set

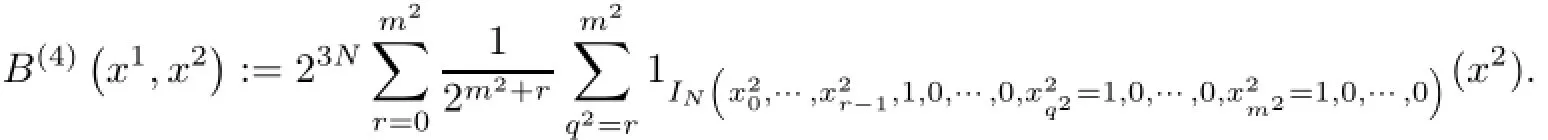
some 0≤r≤m2，r≤q2≤m2.Then we can write

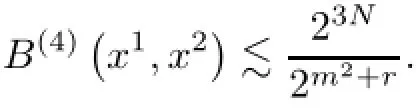
Now，

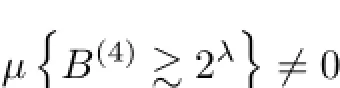
implies that

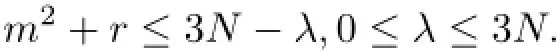
We consider the following two cases.

a）Let 0≤q2≤（3N−λ）/2.Set

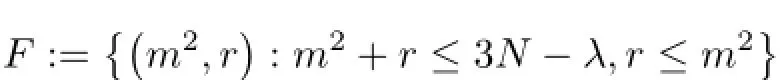
and

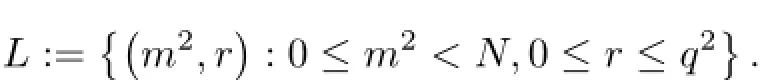
We can write that

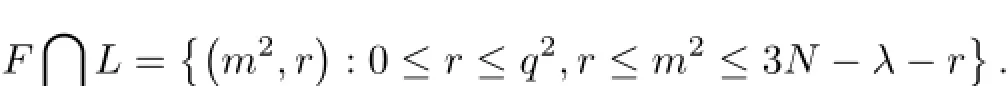
Hence，we obtain

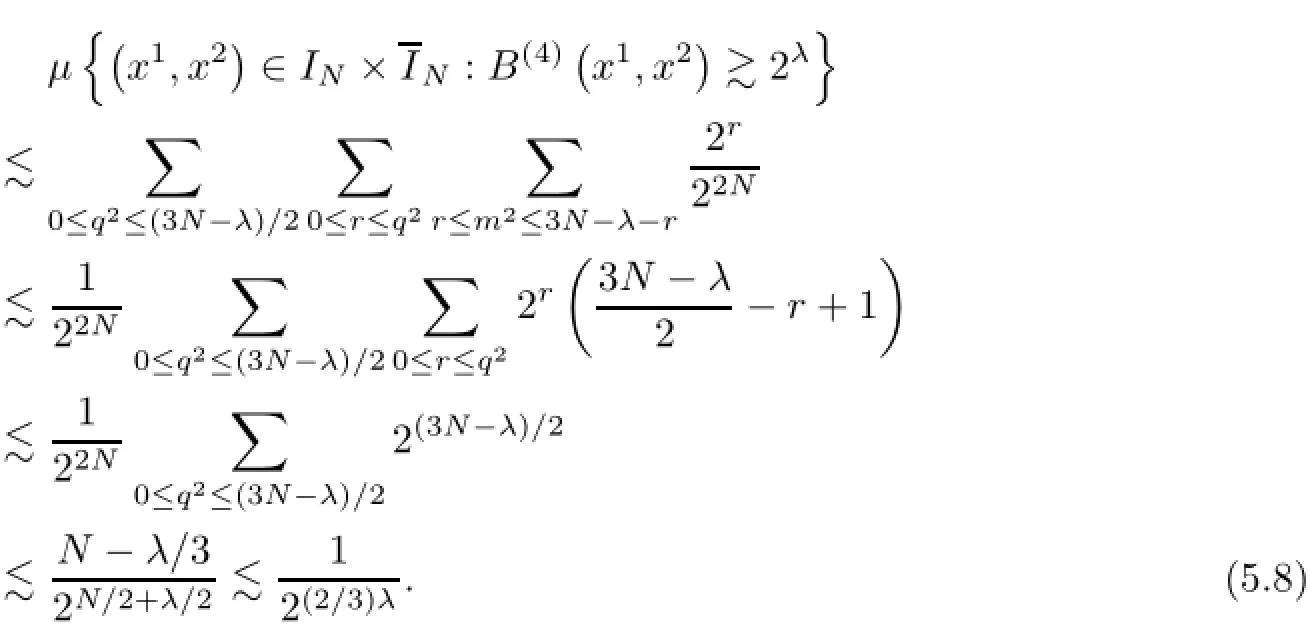
b）Let（3N−λ）/2＜q2≤m2.This implies that

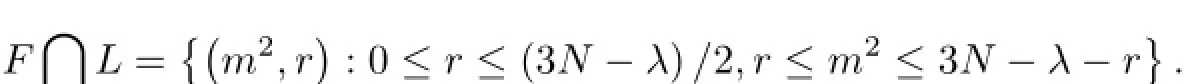
Consequently，

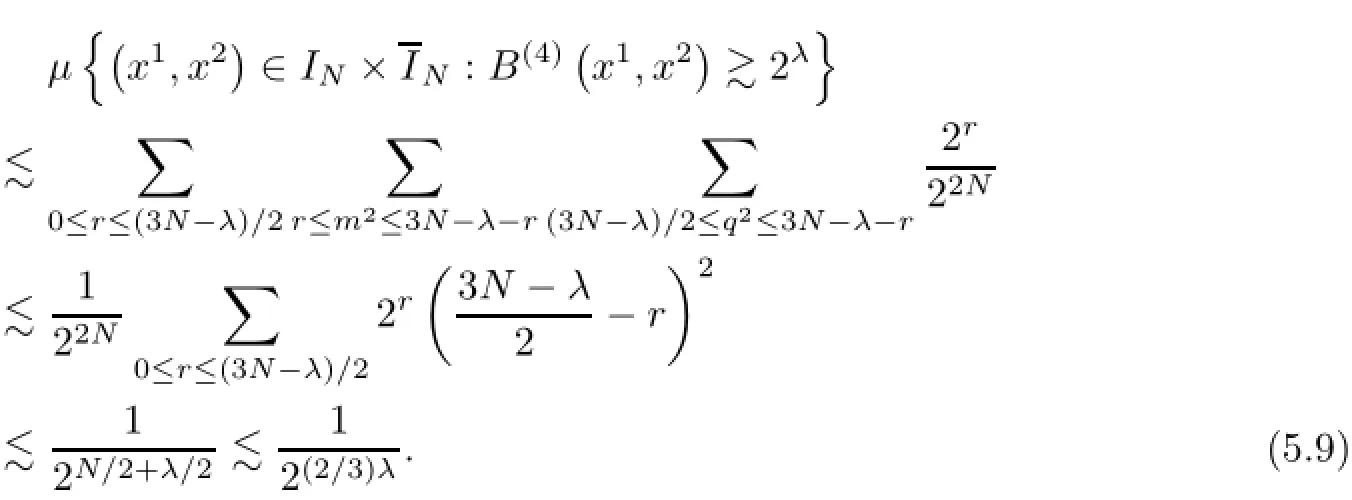
Combining（5.7）-（5.9），we conclude that

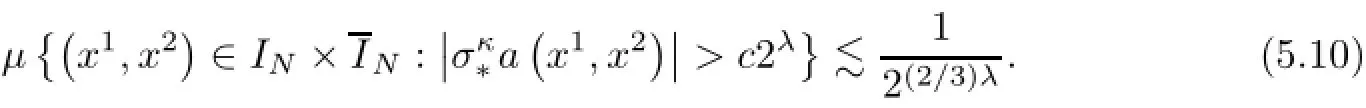
Step 3.Calculating over（KIN）×IN.This case is analogous to Step 2 and we immediately get

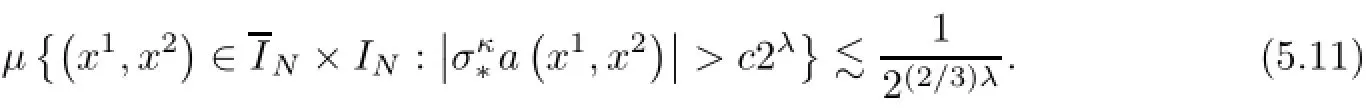
Combining（5.1），（5.5），（5.10），（5.11）and Lemma 4.6，we complete the proof of Theorem 3.1.

References

［1］Weisz F.Summability of multi-dimensional Fourier series and Hardy space.Dordrecht：Kluwer Academic，2002

［2］Goginava U.Marcinkiewicz-Fej´er means of d-dimensional Walsh-Fourier series.Math Anal and Appl，2005，307（1）：206-218

［3］Goginava U.The maximal operator of Marcinkiewicz-Fej´er means of the d-dimensional Walsh-Fourier series. East J Approx，2006，12（3）：295-302

［4］Goginava U.Weak type inequality for the maximal operator of the Marcinkiewicz-Fej´er means of the two-dimensional Walsh-Fourier series.J Approx Theory，2008，154：161-180

［5］Schipp F，Wade W R，Simon P，P´al J.Walsh Series，An Introduction to Dyadic Harmonic Analysis.New York：Adam Hilger Bristol，1990

［6］˘Sneider A A.On series with respect to the Walsh functions with monotone coefficients.Izv Akad Nauk SSSR Ser Math，1948，12：179-192

［7］Schipp F.Pointwise convergence of expansions with respect to certain product systems.Anal Math，1976，2：63-75

［8］Young W S.On the a.e.convergence of Walsh-Kaczmarz-Fourier series.Proc Amer Math Soc，1974，44：353-358

［9］Skvortsov V A.On Fourier series with respect to the Walsh-Kaczmarz system.Anal Math，1981，7：141-150［10］G´at G.On（C，1）summability of integrable functions with respect to the Walsh-Kaczmarz system.Studia Math，1998，130：135-148

［11］Simon P.On the Ces`aro summability with respect to the Walsh-Kaczmarz system.J Approx Theory，2000，106：249-261

［12］Goginava U.The maximal operator of the Fej´er means of the character system of the p-series field in the Kaczmarz rearrangement.Publ Math（Debrecen），2007，71（1/2）：43-65

［13］Tephnadze G.On the maximal operators of Walsh-Kaczmarz-Fej´er means.Period Math Hungar，2013，67（1）：33-45

［14］Tephnadze G.Approximation by Walsh-Kaczmarz-Fej´er means on the Hardy space.Acta Math Sci Ser B Engl Ed，2014，34（5）：1593-1602

［15］Nagy K.On the two-dimensional Marcinkiewicz means with respect to Walsh-Kaczmarz system.J Approx Theory，2006，142：138-165

［16］G´at G，Goginava U，Nagy K.On（Hpq，Lpq）-type inequality of maximal operator of Marcinkiewicz-Fej´er means of double Fourier series with respect to the Kaczmarz system.Math Ineq Appl，2006，9（3）：473-483［17］G´at G，Goginava U，Nagy K.On the Marcinkiewicz-Fej´er means of double Fourier series with respect to the Walsh-Kaczmarz system.Studia Sci Math Hung，2009，6（3）：399-421

［18］Goginava U，Nagy K.On the maximal operator of the Marcinkiewicz-Fej´er means of double Walsh-Kaczmarz-Fourier series.Publ Math（Debrecen），2009，75（1/2）：95-104

［19］Golubov B I，Efimov A V，Skvortsov V A.Series and transformations of Walsh.Moscow，1987（Russian）；English translation.Dordrecht：Kluwer Academic，1991

［20］Weisz F.Martingale Hardy spaces and their applications in Fourier analysis.Berlin：Springer-Verlang，1994［21］Marcinkiewicz J.Sur une methode remarquable de sommation des series doubles de Fourier.Ann Scuola Norm Sup Pisa，1939，8：149-160

［22］Zhizhiashvili L V.Generalization of a theorem of Marcinkiewicz.Izv Akad Nauk USSR Ser Math，1968，32：1112-1122

［23］Weisz F.Convergence of double Walsh-Fourier series and Hardy spaces，Approx Theory and its Appl，2001，17：32-44

［24］G´at G.Convergence of Marcinkiewicz means of integrable functions with respect to two-dimensional Vilenkin systems.Georgian Math J，2004，11（3）：467-478

［25］Nagy K.Some convergence properties of the Walsh-Kaczmarz system with respect to the Marcinkiewicz means.Rendiconti del Circolo Matematico di Palermo，Serie II，2005，76：503-516

October 28，2013；revised June 20，2014.The research was supported by project T´AMOP-4.2.2.A-11/1/KONV-2012-0051 and Shota Rustaveli National Science Foundation grant no.13/06（Geometry of function spaces，interpolation and embedding theorems）.