陈立，何姝琦，董亚莹

（西北大学数学学院，陕西西安710127）

Benny-Luke方程和Phi-4方程的精确行波解

陈立，何姝琦，董亚莹

（西北大学数学学院，陕西西安710127）

运用了tanh-coth展开法，得到了Benny-Luke方程和Phi-4方程的一些新的精确行波解.同时该方法也可用于其他的非线性演化方程.

tanh-coth展开法；Benny-Luke方程；Phi-4方程；精确行波解

1　引言

非线性演化方程被广泛运用于现代科学中，非线性现象出现在科学和工程的许多分支中，例如等离子物理、流体力学、气体动力学、化学反应、生态、固体物理等给人留下深刻印象的研究领域.非线性演化方程经常用来描述孤立波运动，寻求非线性演化方程的精确很重要，因此精确行波解的探索变成非线性物理现象研究的一项重要任务.值得注意的是，观察到不存在独特的方法来解决所有非线性演化方程.由于这个原因，许多方法已被建立，例如Jacobi椭圆函数法［1］、齐次平衡法、MSE方法（Modified Simple Equation Method）［2］、展开法［3［5］、变分法、反散射方法、广田的双线性方法、tanh-coth展开法［69］、不变集方法［10］、不变子空间方法［11］、李群方法等.随着各种求解方法的出现，不但过去难于求解的方程得到解决，而且新的、具有重要物理意义的解不断被发现和应用，呈现出一个层出不穷的势头.

Malfliet首先介绍tanh展开法用于求解非线性偏微分方程，后来此方法被广泛运于求解非线性偏微分方程.Wazwaz将tanh展开法延拓为tanh-coth展开法.本文运用了tanh-coth展开法寻求Benny-Luke方程

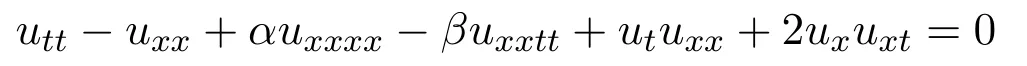
和Phi-4方程的精确行波解.本文安排如下：第2部分，介绍tanh-coth展开法；第3部分，将此方法应用于Benny-Luke方程，并得到该方程的一些新的精确行波解；第4部分，将此方法应用于Phi-4方程，并得到该方程的一些新的精确行波解；第5部分，将Benny-Luke方程和Phi-4方程的精确行波解做了物理解释和图像解释.最后，得知该方法在求解非线性演化方程的精确行波解时非常直观有效.

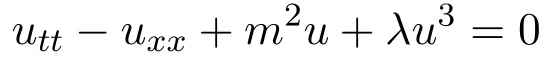
2　tanh-coth展开法

3　求解Benny-Luke方程

Benny-Luke方程是一个近似水波方程，适合在表面张力存在下描述双向水波传播，在物理等方面具有广泛的应用.在这一部分，将运用tanh-coth展开法来研究Benny-Luke方程

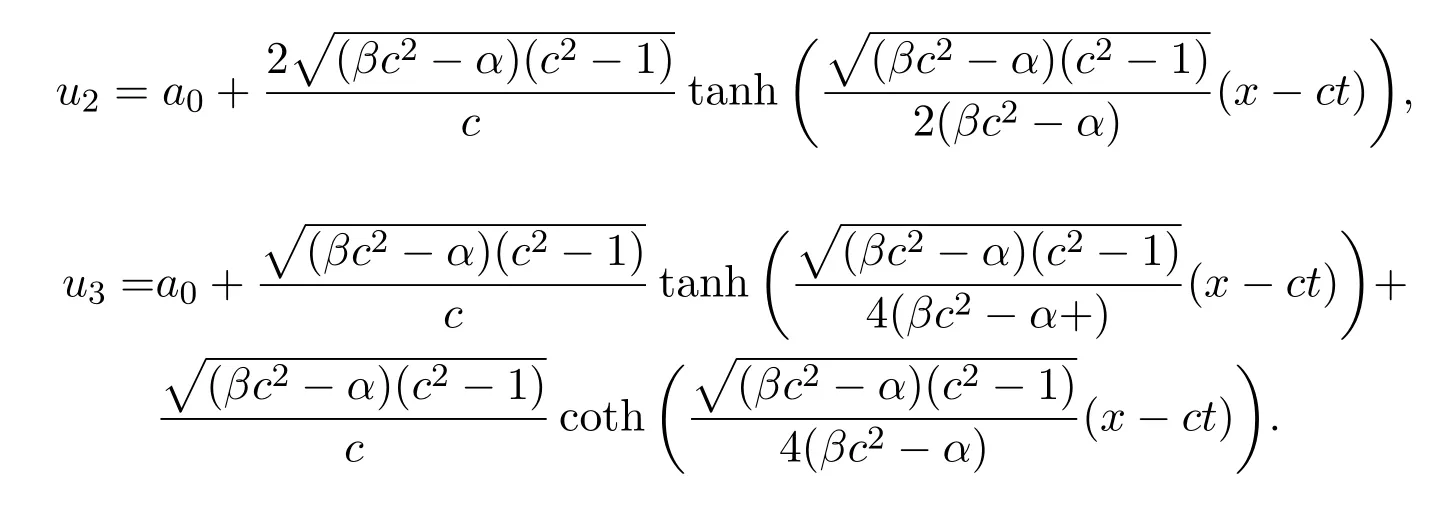
4　求解Phi-4方程

近几十年来，Phi-4方程在核物理和粒子物理占有很重要的地位.在这一部分，将运用tanh-coth展开法来研究Phi-4方程

5　物理解释和图像解释

在这一部分，将给出Benny-Luke方程和Phi-4方程的精确行波解的物理解释和图像解释.

本文第三部分运用tanh-coth展开法得到Benny-Luke方程的精确行波解u1，u2，u3.当取a0=1，α=1，β=1，（-10≤x≤10），（0≤t≤10）时，u1的图像如图1所示，图像1表示Benney-Luke方程的扭结型行波解，u2的图像如图2所示，图像2表示Benney-Luke方程的奇异扭结型行波解，u3的图像与u2的图像类似.

本文第四部分运用tanh-coth展开法得到Phi-4方程的精确行波解u1，u2，u3，u4，u5，u6，u7，u8.当取λ=-1.2，m=139，c=2，（-10≤x≤10），（0≤t≤10）时，u1的图像如图3所示，图像3表示Phi-4方程的多重周期解，u3的图像如图4所示，图像4表示Phi-4方程的奇异扭结型行波解，u2的图像与u1的图像类似，u4，u5，u6，u7，u8的图像与u3的图像类似.

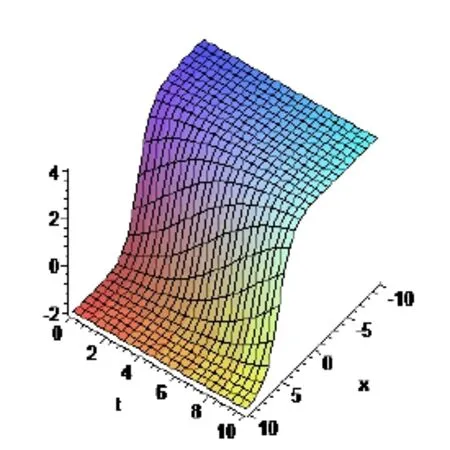
图1　Benny-Luke方程的解u1

图2　Benny-Luke方程的解u2

图3　Phi-4方程的解u1

图4　Phi-4方程的解u3

6　总结

本文运用tanh-coth展开法求解出Benny-Luke方程和Phi-4方程的一些新的行波解，从而丰富了方程的结果，有助于对方程所描述的物理现象进一步了解和研究.同时，这种方法也能够求解其他的非线性演化方程，也可以将双曲函数换成三角函数，同样能够得到一些方程的行波解.

［1］Ali A T.New generalized Jocobi ellipic function rational expansion method［J］.Comput.Appl.Math.，2011，235：4117-4127.

［2］Akter J，Akbar M A.Exact solutions to the Benny-Luke equations and the Phi-4 equations by using modified simple equation method［J］.Results in Physics，2015，5：125-130.

［3］Wang M，Li X，Zhang J.The（G′/G）-expansion method and travelling wave solutions of nonlinear evolution equations in mathematical physics［J］.Phys.Lett.A，2008，372：417-423.

［4］Zayed E M E，Gepreel K A.The（G′/G）-expansion method for finding the travelling wave solutions of nonlinear evolution equations in mathematical physics［J］.Math.Phys.，2009，50：013502-013514.

［5］Mohyud S T，Yildirim A，Saruaydin S.Numerical solitons of the improved Boussinesq equation［J］.Int.J.Numer.Method H.，2011，21：822-827.

［6］Malfliet W.Solitary wave solutions of nonlinear wave equations［J］.Am.J.Phys.，1992，60（7）：650-654.

［7］Wazwaz A M.New travelling wave solutions to the Boussinesq and the Klein-Gordon Equations［J］.Commun.Nonlinear Sci.Numer.Simulat.，2008，13：889-901.

［8］Wazwaz A M，Mona S M.A variety of exaction travelling wave solutions for the（2+1）-dimensional Boiti-Leon-Pempinelli equation［J］.Appl.Math.Comput.，2010，217：1484-1490.

［9］Wazwaz A M.The tanh-coth method for solitons and kink solutions for nonlinear parabolic equations［J］.Appl.Math.Comput.，2007，188：1467-1475.

［10］Dong Y Y，Zhang S L，Li S B.Invariant sets and solutions to a class of wave equations［J］.Appl.Math.Comput.，2015，253：369-376.

［11］Qu G Z，Zhang S L，Li Y L.Third-order nonlinear differential operators preserving invariant subspaces of maximal dimension［J］.Chin.Phys.B，2014，23：110202.

New travelling wave solutions to the Benny-Luke equation and the Phi-4 equations

Chen Li，He Shuqi，Dong Yaying

（College of Mathematics，Northwest University，Xi′an710127，China）

In this work，many new travelling wave solutions are established for the Benny-Luke equation and the Phi-4 equations.The tanh-coth method is used to generate these new solutions.The approach is also applicable to a large variety of nonlinear evolution equations.

the tanh-coth method，the Benny-Luke equation，the Phi-4 equations，travelling wave solutions

O175.29

A

1008-5513（2015）06-0604-07

10.3969/j.issn.1008-5513.2015.06.008

2015-06-16.

国家自然科学基金（11371293）.

陈立（1989-），硕士生，研究方向：偏微分方程.

2010 MSC：35C07